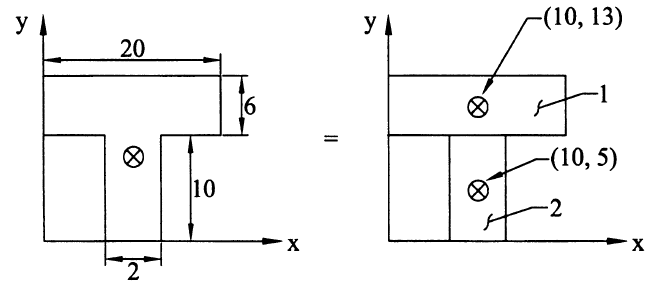
Find the centroid, relative to x and y, for the cross section shown in Fig. 3.25.

Question

Accepted Answer

It is easiest to formulate these solutions in tabular form. Hence, note that

[latex]\overline{y}_{i}A_{i}[/latex]	[latex]\overline{x}_{i}A_{i}[/latex]	Area
13(120)	10(120)	20(6)=120	Part 1
5(20)	10(20)	2(10)=20	Part 2

Therefore,FIGURE 3.25 Compute the first moment of area of this composite section.

[latex]\overline{x}=\frac{\sum{\overline{x}_{i}A_{i} } }{\sum{A_{i}} }=\frac{10(120)+10(20)}{120+20}=10,[/latex]

[latex]\overline{y}=\frac{\sum{\overline{y}_{i}A_{i} } }{\sum{A_{i}} }=\frac{13(120)+5(20)}{120+20}=11.86[/latex]

Note, too, that we could obtain the same result by computing values for a rectangular area [latex]20 imes 16[/latex] and then subtracting out small rectangular areas to yield the T-shape, namely

[latex]\overline{y}_{i}A_{i}[/latex]	[latex]\overline{x}_{i}A_{i}[/latex]	Area
8(320)	10(320)	20(16)=320	Part 1
5(90)	4.5(90)	9(10)=90	Part 2
5(90)	15.5(90)	9(10)=90	Part 3

whereby

[latex]\overline{x}=\frac{\sum{\overline{x}_{i}A_{i} } }{\sum{A_{i}} }=\frac{10(320)-4.5(90)-15.5(90)}{320-90-90}=10,[/latex]

[latex]\overline{y}=\frac{\sum{\overline{y}_{i}A_{i} } }{\sum{A_{i}} }=\frac{8(320)-5(90)-5(90)}{320-90-90}=11.86.[/latex]

which is the same as found above.

Question A3.3: Find the centroid, relative to x and y, for the cross sectio...

Related Answered Questions