Question 4.7.3: A jig applied to sizing coal contains a screen that reciproc......

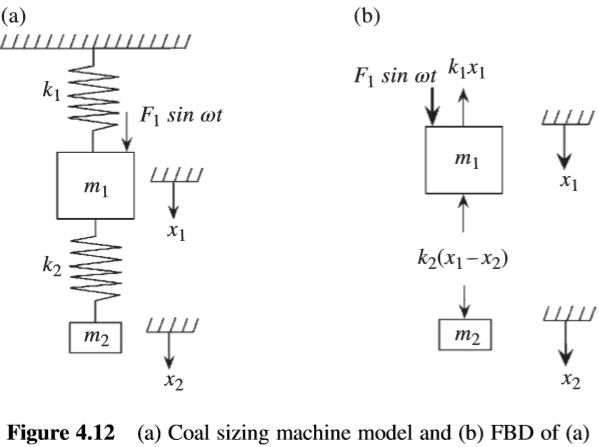
The conceptual model of this 2-dof system and its FBD have already been presented in Figure 4.12. Fundamental frequency of the jig is Ω_1 = \sqrt{ \frac{k_{1}}{m_{1}} }  = 20(2π) rad /s, and mass of the jig m_{1} = 200kg.
Therefore, the stiffness, k_{1} = [20( 2 π )]² × 200 N/ m = 3.1583 × 10^{6}N/ m.
With reference to Figure 4.12, the present 2-dof system has the amplitudes of the displacement responses defined by Equations (4.21a) and (4.21b). Thus,

X_{1} =  \frac{(k_{22}  −   m_{2}ω²)  F_{1}}{m_{1}m_{2}  ( ω_{1}²  –  ω²) ( ω_{2}²  –  ω²)}  ,                                        (4.21 a)

X_{2} =  \frac{-  k_{21}  F_{1}}{m_{1}m_{2}  ( ω_{1}²  –  ω²) ( ω_{2}²  –  ω²)}  .                                        (4.21 b)

a. For the jig frame to have no steady-state vibration when the absorber is attached, X_{1}  = 0. From Equation (4.21a), one requires that k_{22}   =  m_{2}ω².
The equations of motion for this system are given by

\begin{bmatrix} m_{1} & 0 \\ 0 & m_{2} \end{bmatrix}   \begin{pmatrix} \ddot{x_{1} }\\ \ddot{ x_{2}} \end{pmatrix}  +    \begin{bmatrix} (k_{1}  +   k_{2})  &    –   k_{2} \\ –   k_{2} &  k_{2} \end{bmatrix}   \begin{pmatrix} x_{1} \\  x_{2} \end{pmatrix}  =   \begin{pmatrix} 0 \\ 0 \end{pmatrix}.

Hence, k_{22}   =  k_{2}  =  m_{2}ω². But the absorber mass m_{2} = 30. 5 kg and the applied frequency ω = 400 rpm.

Therefore, ω = 400 (2π)/(60) rad/s  =  41.8879  rad/s..
This gives the absorber stiffness,

k_{2}  =  m_{2}ω² = 30.5 × 41.8879² N /m
=30.5×41.8879² N/ m = 53515.1831 N /m.

b. Substituting the above parameters, m_{1} =  200  kg,   m_{2}    =  30.5  kg,   k_{1}  =   3.1583   ×   10^{6}  N/m,   k_{2}   = 53515.1831  N/m, into Equations (A2a) and (A2b) of Appendix 4A, one obtains

ω_{1} =  41.54  rad/s,     ω_{2} = 126.84 rad/s.

These are the two required natural frequencies of the system.