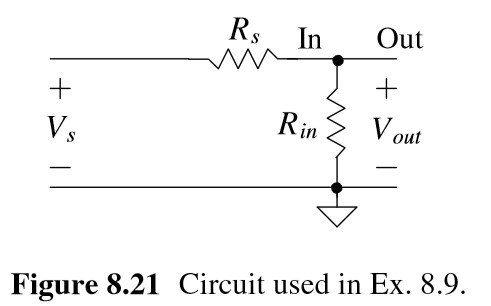
Question 8.9: Calculate the input-referred noise, F, and SNRs for the circ......

Let’s begin by adding the noise voltage spectral density to the circuit, Fig. 8.22a. The output noise PSD is

To determine $V_{o n o i s e,R M S}$, we integrate this PSD over the bandwidth of interest B or

Noting our gain A (= $V_{_{o u t}}/V_{i n}$ not $V_{_{o u t}}/V_{s}$) is one, we can use the model shown in Fig. 8.22b. To determine the input-referred noise sources, we can use Eq. (8.32) and the results in Ex. 8.7. To determine $\textstyle V_{i n o i s e,R M S}$, we short the input to ground ($R_{s}=0$ in Fig. 8.21 and the equation above), Fig. 8.22c, and equate the circuit output to $\textstyle V_{o n o i s e,R M S}$. This gives

$V_{o n o i s e,R M S}^{2}=4k T R_{s}B\cdot\left(\frac{A R_{i n}}{R_{s}+R_{i n}}\right)^{2}+I_{i n o i s e,R M S}^{2}\cdot\left(\frac{A R_{s}R_{i n}}{R_{s}+R_{i n}}\right)^{2}+V_{i n o i s e,R M S}^{2}\cdot\left(\frac{A R_{i n}}{R_{s}+R_{i n}}\right)^{2}$  (8.32)

To determine $\textstyle I_{i n o i s e,R M S}$, we open the input ($R_{s}=∞$), Fig. 8.22d, and equate the Rs circuit’s output to $\textstyle V_{o n o i s e,R M S}$ (from the equation above). This gives

The input SNR is given in Eq. (8.29). The output SNR, Fig. 8.22e, is

$S N R_{i n}=\frac{V_{s,R M S}^{2}\cdot\left[\frac{R_{i n}}{R_{i n}+R_{s}}\right]^{2}}{4k T R_{s}B\cdot\left[\frac{R_{i n}}{R_{i n}+R_{s}}\right]^{2}}=\frac{V_{s,R M S}^{2}}{4k T R_{s}B}$       (8.29)

$\mathrm{SN}R_{o u t}={\frac{V_{s,R M S}^{2}\cdot\left[{\frac{R_{i n}}{R_{s^{+}R_{i n}}}}\right]^{2}}{V_{o n o i s e,R M S}^{2}}}={\frac{V_{s,R M S}^{2}}{4k T B\cdot R_{s}(1+R_{s}/R_{i n})}}$        (8.40)

The noise factor is then

$F=1+\frac{R_{s}}{R_{i n}}$                    (8.41)

To minimize the NF, we can decrease $R_{s}$ or increase $R_{in}$. Decreasing $R_{s}$ causes $SNR_{in}$ and $SNR_{out}$ to increase, as seen in Eqs. (8.29) and (8.40). At the same time, increasing $R_{in}$ causes $SNR_{out}$ to move towards $SNR_{in}$, Eq. (8.40), resulting in F moving towards 1.