Question 2.SP.2: Two tugboats are pulling a barge. If the resultant of the fo......

MODELING: You can use the parallelogram law or the triangle rule to solve part (a). For part (b), use a variation of the triangle rule.

ANALYSIS: a. Tension for α = 45°.

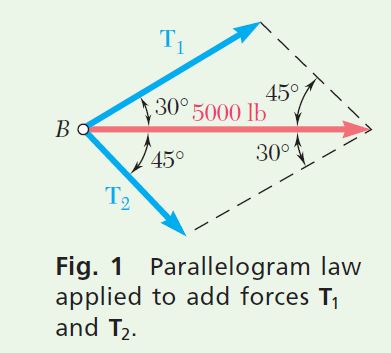
Graphical Solution. Use the parallelogram law. The resultant (the diagonal of the parallelogram) is equal to 5000 lb and is directed to the right. Draw the sides parallel to the ropes (Fig. 1). If the drawing is done to scale, you should measure

T_1=3700 ~\mathrm{lb} \quad T_2=2600 ~\mathrm{lb}

Trigonometric Solution. Use the triangle rule. Note that the triangle in Fig. 2 represents half of the parallelogram shown in Fig. 1. Using the law of sines,

\frac{T_1}{\sin 45^{\circ}}=\frac{T_2}{\sin 30^{\circ}}=\frac{5000 ~\mathrm{lb}}{\sin 105^{\circ}}

With a calculator, compute and store the value of the last quotient. Multiply this value successively by sin 45° and sin 30°, obtaining

T_1=3660 ~\mathrm{lb} \quad T_2=2590 ~\mathrm{lb}

b. Value of α for Minimum T_2. To determine the value of α for which the tension in rope 2 is minimum, use the triangle rule again. In Fig. 3, line 1-1′ is the known direction of \mathbf{T}_1. Several possible directions of \mathbf{T}_2 are shown by the lines 2-2′. The minimum value of T_2 occurs when \mathbf{T}_1 \text { and } \mathbf{T}_2 are perpendicular (Fig. 4). Thus, the minimum value of T_2 is

T_2=(5000 ~\mathrm{lb}) \sin 30^{\circ}=2500 ~\mathrm{lb}

Corresponding values of T_1 \text { and } \alpha are

\begin{aligned}T_1 & =(5000 ~\mathrm{lb}) \cos 30^{\circ}=4330 ~\mathrm{lb} \\\alpha & =90^{\circ}-30^{\circ} \quad \alpha=60^{\circ}\end{aligned}

REFLECT and THINK: Part (a) is a straightforward application of resolving a vector into components. The key to part (b) is recognizing that the minimum value of T_2 \text { occurs when } \mathbf{T}_1 \text { and } \mathbf{T}_2 are perpendicular.