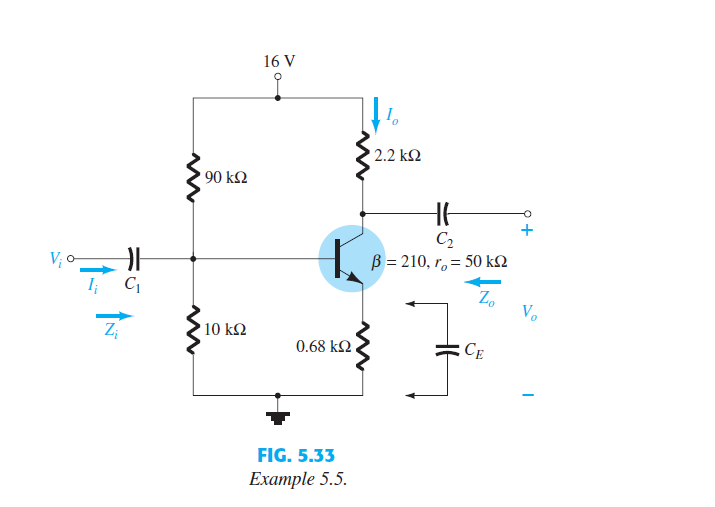
Question 5.5: For the network of Fig. 5.33 (with C E unconnected), determi...
For the network of Fig. 5.33 (with C_E unconnected), determine (using
appropriate approximations):
a. r_e .
b. Z_i .
c. Z_o .
d. A_v
a. Testing \beta R_{E}>10 R_{2}.
(210)(0.68 kΩ) > 10(10 kΩ)
142.8 kΩ >100 kΩ (satisfied)
we have
V_{B}=\frac{R_{2}}{R_{1}+R_{2}} V_{C C}=\frac{10 k \Omega}{90 k \Omega+10 k \Omega}(16 V )=1.6 VV_{E}=V_{B}-V_{B E}=1.6 V -0.7 V =0.9 V
I_{E}=\frac{V_{E}}{R_{E}}=\frac{0.9 V }{0.68 k \Omega}=1.324 mA
r_{e}=\frac{26 mV }{I_{E}}=\frac{26 mV }{1.324 mA }= 1 9 . 6 4 \Omega
b. The ac equivalent circuit is provided in Fig. 5.34 . The resulting configuration is different from Fig. 5.30 only by the fact that now
R_{B}=R^{\prime}=R_{1} \| R_{2}=9 k \OmegaThe testing conditions of r_{o} \geq 10\left(R_{C}+R_{E}\right) and r_{o} \geq 10 R_{C} are both satisfied. Using the appropriate approximations yields
Z_{b} \cong \beta R_{E}=142.8 k \OmegaZ_{i}=R_{B}\left\|Z_{b}=9 k \Omega\right\| 142.8 k \Omega
= 8.47 kΩ
c. Z_{o}=R_{C}=2.2 k \Omega
d. A_{v}=-\frac{R_{C}}{R_{E}}=-\frac{2.2 k \Omega}{0.68 k \Omega}=- 3 . 2 4
Related Answered Questions
Question: 5.23
Verified Answer:
The common-base hybrid parameters are derived from...
Question: 5.22
Verified Answer:
Now that the basic equations for each quantity hav...
Question: 5.18
Verified Answer:
I_{B_{1}}=\frac{18 V -0.7 V }{2 M \Omega+(1...
Question: 5.14
Verified Answer:
a. For the emitter-follower configuration, the loa...
Question: 5.13
Verified Answer:
a. Eq. (5.89): A_{v_{L}}=\frac{R_{L}}{R_{L}...
Question: 5.9
Verified Answer:
a. I_{B}=\frac{V_{C C}-V_{B E}}{R_{F}+\beta...
Question: 5.21
Verified Answer:
a. Z_{i}=R_{E}\left\|h_{i b}=2.2 k \Omega\r...
Question: 5.20
Verified Answer:
a. Z_{i}=R_{B}\left\|h_{i e}=330 k \Omega\...
Question: 5.19
Verified Answer:
a. r_{e}=\frac{26 mV }{I_{E}}=\frac{26 mV }...
Question: 5.17
Verified Answer:
\beta_{D}=\beta_{1} \beta_{2}=(50)(100)= 5 ...