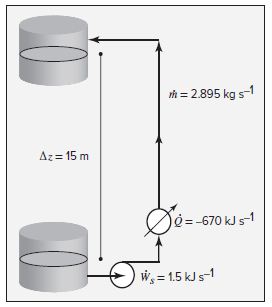
Question 2.14: Water at 90°C is pumped from a storage tank at a rate of 3 L...

This is a steady-state, steady-flow process for which Eq. (2.31) applies. The initial and final velocities of water in the storage tanks are negligible, and the term Δu^{2}/2

can be omitted. All remaining terms are expressed in units of kJ·kg^{−1} . At 90°C the density of water is 0.965  kg·L^{−1} and the mass flow rate is:

\Delta H + \frac{\Delta u^2}{2} + g \Delta z=Q+W_s                 (2.31)

\dot{m}=(3)(0.965)=  2.895  kg⋅s^{-1}

For the heat exchanger,

Q = − 670 / 2.895 = − 231.4  kJ⋅ kg^{−1}

For the shaft work of the pump,

W_{s}=1.5 / 2.895 = 0.52  kJ⋅ kg ^{−1}

If g is taken as the standard value of 9.8 m·s^{−2} , the potential-energy term is:

g\Delta z= (9.8) (15)= 147  m^{2} .s^{-2}= 147  J⋅ kg^{−1} = 0.147  kJ⋅ kg^{−1}

The steam-table value for the enthalpy of liquid water at 90°C is:

H_{1} = 376.9  kJ⋅ kg ^{−1}

Thus,

ΔH = H_{2}   −  ​H_{1} = H_{2}  −  376.9 = − 231.0

and

H_{2}   = 376.9  −  231.0 = 145.9  kJ⋅ kg ^{−1}

The temperature of water having this enthalpy is found from the steam tables:

t = 34.83° C

In this example, W_{s} and gΔz are small compared with Q, and for practical purposes could have been neglected.