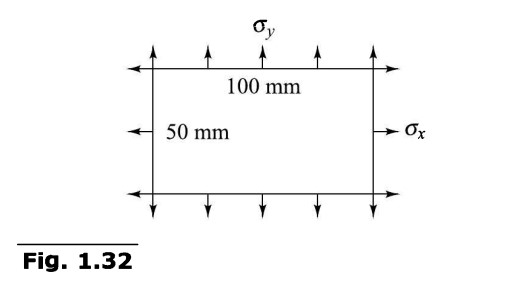
Question 1.22: A thin rectangular steel plate 100 mm by 50 mm undergoes def...
A thin rectangular steel plate 100 mm by 50 mm undergoes deformations 0.05 mm and 0.01 mm in x and y directions due to stresses a,\sigma _x and \sigma _y as shown in Fig. 1.32. Determine the magnitude of \sigma _x and \sigma _y if modulus of elasticity is 200 GPa and Poisson’s ratio is 0.3.
It is plane stress problem where,
\varepsilon_x=\frac{0.05}{100}=0.005 mm / mm , and
\varepsilon_y=\frac{0.01}{50}=0.0002 mm / mmSubstituting in Eq. (1.231),
\sigma_x =\frac{E}{1-\mu^2}\left(\varepsilon_x+\mu \varepsilon_y\right)=\frac{200000}{1-0.3^2}(0.0005+0.3 \times 0.0002) =123.07 MPa
\sigma_y =\frac{E}{1-\mu^2}\left(\varepsilon_y+\mu \varepsilon_x\right)
=\frac{200000}{1-0.3^2}(0.0002+03 \times 0.0005) =76.92 MPa
Related Answered Questions
Question: 1.19
Verified Answer:
Equilibrium condition: For static eq-uilibrium of ...
Question: 1.18
Verified Answer:
Solution Equilibrium condition: The forces in sect...
Question: 1.17
Verified Answer:
Area of steel bars,
A_s = 4 \times \left(\p...
Question: 1.16
Verified Answer:
First consider the external load alone. Equilibriu...
Question: 1.30
Verified Answer:
Static deflection of the beam,
\sigma_{s t}...
Question: 1.28
Verified Answer:
Using Eq. (1.28)
\sigma _{\text{max}}^2 -2\...
Question: 1.29
Verified Answer:
The deflection at midspan due to statically applie...
Question: 1.27
Verified Answer:
Using Eq. 1.28,
\sigma _{\text{max}}^2 -2\f...
Question: 1.26
Verified Answer:
An element ABCD shown in Fig. 1.35, deforms to [la...
Question: 1.25
Verified Answer:
Figure E. 1.34 shows an element under radial stres...