Solving an Equation Involving Inverse Trigonometric Functions Solve [latex]\cos^{-1} x = \sin^{-1} \frac{1}{2}[/latex] .

Let [latex]\sin^{-1} \frac{1}{2} = u[/latex]. Then [latex]\sin u = \frac{1}{2}[/latex] , and for u in quadrant I we have the following. [latex]\cos^{-1} x = \sin^{-1} \frac{1}{2}[/latex] Original equation [latex]\cos^{-1} x = u[/latex] Substitute. [latex]\cos u = x[/latex] Alternative form Sketch a triangle and label it using the facts that u is in quadrant I and [latex]\sin u = \frac{1}{2}[/latex]. See Figure 39. Because [latex]x = \cos u[/latex], we have [latex]x = \frac{\sqrt{3}}{2}[/latex] . The solution set is {[latex] \frac{\sqrt{3}}{2}[/latex] } .

Question 7.7.3: Solving an Equation Involving Inverse Trigonometric Function...

Precalculus

Solving an Equation Involving Inverse Trigonometric Functions

Solve $\cos^{-1} x = \sin^{-1} \frac{1}{2}$ .

The blue check mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.

Let $\sin^{-1} \frac{1}{2} = u$ . Then $\sin u = \frac{1}{2}$ , and for u in quadrant I we have the following.

$\cos^{-1} x = \sin^{-1} \frac{1}{2}$ Original equation

$\cos^{-1} x = u$ Substitute.

$\cos u = x$ Alternative form

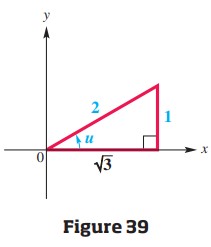
Sketch a triangle and label it using the facts that u is in quadrant I and $\sin u = \frac{1}{2}$ . See Figure 39. Because $x = \cos u$ , we have $x = \frac{\sqrt{3}}{2}$ . The solution set is { $\frac{\sqrt{3}}{2}$ } .