Question 18.8: Universal Motor Torque Expression Compute an expression for ...
Universal Motor Torque Expression
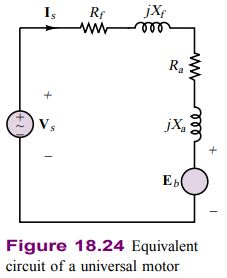
Compute an expression for the average torque generated by a universal motor, based on the circuit diagram of Figure 18.24.
Learn more on how we answer questions.
Known Quantities: Circuit model of motor.
Find: Expression for average torque, T_{\text {ave }}.
Assumptions: The motor operates in the linear region of the magnetization curve.
Analysis: With reference to Chapter 17, we know that the flux produced in a series motor by the series current i_{S}(t) is \phi=k_{S} i_{S}(t). The instantaneous torque produced by the machine is given by the expression
T(t)=k_{T} \phi(t) i_{s}(t)
If the source waveform has period \tau=2 \tau / \omega, we can calculate the average power by integrating the instantaneous torque over one period:
T_{\mathrm{ave}}(t)=\frac{\omega}{2 \pi} \int_{0}^{2 \pi / \omega} k_{T} k_{S} i_{S}^{2}\left(t^{\prime}\right) d t^{\prime}=\frac{\omega}{2 \pi} \int_{0}^{2 \pi / \omega} k_{T} k_{S} I_{S}^{2}\left(\sin ^{2} \omega t^{\prime}\right) d t^{\prime}=\frac{1}{2} k_{T} k_{S} I_{S}^{2}
where I_{S} is the rms value of the (series) armature current
Comments: The series motor can produce a nonzero average torque when excited by an AC current because of the quadratic nature of the instantaneous torque. A PM DC machine, which has a linear torque-current relationship, would generate zero average torque if driven from an AC supply.