Question 6.SP.4: In the frame shown, members ACE and BCD are connected by a p......

STRATEGY: Follow the general procedure discussed in this section. First treat the entire frame as a free body, which will enable you to find the reactions at A and B. Then, dismember the frame and treat each member as a free body, which will give you the equations needed to find the force at C.

MODELING and ANALYSIS: Because the external reactions involve only three unknowns, compute the reactions by considering the free-body diagram of the entire frame (Fig. 1).

+↑\Sigma F_{y}=0\colon\quad A_{y}-480\,\mathrm{N}=0\qquad\qquad A_{y}=+480\,\mathrm{N}\qquad\qquad\mathrm{A_{y}=480\,\mathrm{N}} ↑

+↺ΣM_{A} = 0:   − (480 N)(100 mm) + B(160 mm) = 0

B = +300 N                   B = 300 N →

\underrightarrow{+}\Sigma F_{x}=0\colon \qquad B+A_{x}=0

300\,\mathrm{N}+A_{x}=0\qquad\qquad A_{x}=-300\,\mathrm{N}\qquad\qquad\mathrm{A_{x}=300\,N} ←

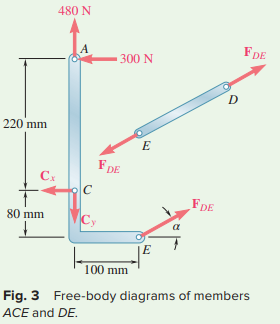
Now dismember the frame (Fig. 2 and Fig. 3). Because only two members are connected at C, the components of the unknown forces acting on ACE and BCD are, respectively, equal and opposite. Assume that link DE is in tension (Fig. 3) and exerts equal and opposite forces at D and E, directed as shown.

Free Body: Member BCD. Using the free body BCD (Fig. 2), you can write and solve three equilibrium equations:

+⟳ΣM_{C} = 0:

(F_{D E}\sin\alpha)(250\,\mathrm{mm})+(300\,\mathrm{N})(60\,\mathrm{mm})+(480\,\mathrm{N})(100\,\mathrm{mm})=0

F_{D E}=-561~\mathrm{{N}}{~}{~}\qquad\qquad\qquad\qquad F_{D E}=561~\mathrm{{N}}\ C  ◂

\underrightarrow{+}\Sigma F_{x}=0\colon \qquad C_{x}-F_{D E}\cos\alpha+300\,\mathrm{N}=0

C_{x}-(-561\ \mathrm{N})\cos28.07^{\circ}+300\ \mathrm{N}=0\qquad C_{x}=-795\ \mathrm{N}

+↑ΣF_{y} = 0\colon \qquad C_{y}-F_{D E}\sin\alpha-480\,\mathrm{N}=0

C_{y}-(-561\mathrm{~N})\mathrm{sin}\,28.07^{\circ}-480\mathrm{~N}=0\qquad\quad C_{y}=+216\mathrm{~N}

From the signs obtained for C_{x}~and~C_{y}, the force components C_{x}~and~C_{y} exerted on member BCD are directed to the left and up, respectively. Thus, you have

\mathbf{C}_{x}=795\;\mathbf{N}\leftarrow,\mathbf{C}_{y}=216\;\mathbf{N}\uparrow   ◂

REFLECT and THINK: Check the computations by considering the free body ACE (Fig. 3). For example,

+↺ΣM_{A}=(F_{D E}\cos\alpha)(300\,\mathrm{mm})+(F_{D E}\sin\alpha)(100\,\mathrm{mm})-C_{x}(220\,\mathrm{mm})

\qquad\qquad= (−561 cos α)(300) + (−561 sin α)(100) − (−795)(220) = 0