Question 11.CA.16: A load P is supported at B by three rods of the same materia......
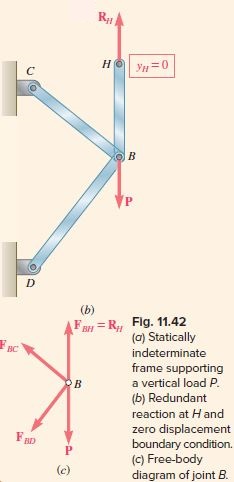
A load P is supported at B by three rods of the same material and the same cross-sectional area A (Fig. 11.42a). Determine the force in each rod.

Learn more on how do we answer questions.
The structure is statically indeterminate to the first degree. The reaction at H is choosen as the redundant. Thus rod BH is released from its support at H. The reaction R _H is now considered to be an unknown load (Fig. 11.42b) and will be determined under the condition that the deflection y _H of point H must be zero. By Castigliano’s theorem, y_H=\partial U / \partial R_H , where U is the strain energy of the three-rod system under load P and the redundant reaction R _H .
Recalling Eq. (11.59),
x_j=\frac{\partial U}{\partial P_j}=\sum_{i=1}^n \frac{F_i L_i}{A_i E} \frac{\partial F_i}{\partial P_j} (11.59)
y_H=\frac{F_{B C}(B C)}{A E} \frac{\partial F_{B C}}{\partial R_H}+\frac{F_{B D}(B D)}{A E} \frac{\partial F_{B D}}{\partial R_H}+\frac{F_{B H}(B H)}{A E} \frac{\partial F_{B H}}{\partial R_H} (1)
Note that the force in rod BH is equal to R_H , or
F_{B H}=R_H (2)
Then, from the free-body diagram of pin B (Fig. 11.42c),
F_{B C}=0.6 P-0.6 R_H \quad F_{B D}=0.8 R_H-0.8 P (3)
Differentiating with respect to R_H the force in each rod gives
\frac{\partial F_{B C}}{\partial R_H}=-0.6 \quad \frac{\partial F_{B D}}{\partial R_H}=0.8 \quad \frac{\partial F_{B H}}{\partial R_H}=1 (4)
Substituting from Eqs. (2), (3), and (4) into Eq. (1) and noting that the lengths BC, BD, and BH are equal to 0.6l, 0.8l, and 0.5l, respectively,
\begin{aligned} y_H=\frac{1}{A E}[ & \left(0.6 P-0.6 R_H\right)(0.6 l)(-0.6) \\ & \left.+\left(0.8 R_H-0.8 P\right)(0.8 l)(0.8)+R_H(0.5 l)(1)\right] \end{aligned}
Setting y_H=0 gives
1.228 R_H-0.728 P=0
and solving for R_H :
R_H=0.593 P
Carrying this value into Eqs. (2) and (3), the forces in the three rods are
F_{B C}=+0.244 P \quad F_{B D}=-0.326 P \quad F_{B H}=+0.593 P