Question 24.5: A Plane of Charge Find the electric field due to an infinite......

Conceptualize Notice that the plane of charge is infinitely large. Therefore, the electric field should be the same at all points equidistant from the plane.

Categorize Because the charge is distributed uniformly on the plane, the charge distribution is symmetric; hence, we can use Gauss’s law to find the electric field.

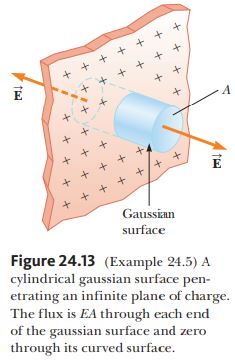
Analyze By symmetry, \overrightarrow{\mathbf{E}} must be perpendicular to the plane at all points. The direction of \overrightarrow{\mathbf{E}} is away from positive charges, indicating that the direction of \overrightarrow{\mathbf{E}} on one side of the plane must be opposite its direction on the other side as shown in Figure 24.13. A gaussian surface that reflects the symmetry is a small cylinder whose axis is perpendicular to the plane and whose ends each have an area A and are equidistant from the plane. Because \overrightarrow{\mathbf{E}} is parallel to the curved surface-and therefore perpendicular to \overrightarrow{d \mathbf{A}} everywhere on the surfacecondition (3) is satisfied and there is no contribution to the surface integral from this surface. For the flat ends of the cylinder, conditions (1) and (2) are satisfied. The flux through each end of the cylinder is E A; hence, the total flux through the entire gaussian surface is just that through the ends, \Phi_{E}=2 E A.

Write Gauss’s law for this surface, noting that the enclosed charge is q_{\text {in }}=\sigma A :

\Phi_{E}=2 E A=\frac{q_{\text {in }}}{\epsilon_{0}}=\frac{\sigma A}{\epsilon_{0}}

Solve for E :

E=\frac{\sigma}{2 \epsilon_{0}}       (24.8)

Finalize Because the distance from each flat end of the cylinder to the plane does not appear in Equation 24.8, we conclude that E=\sigma / 2 \epsilon_{0} at any distance from the plane. That is, the field is uniform everywhere.