Question 5.8: Consider the following histogram, which represents the neutr......
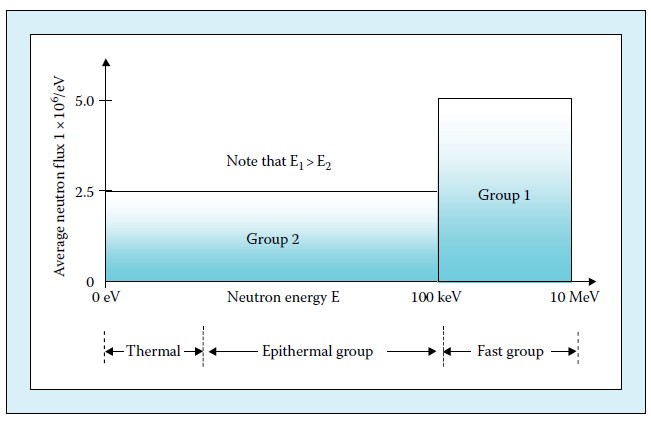
Consider the following histogram, which represents the neutron energy spectrum in a thermal water reactor:
If the Y axis shows the average neutron flux <ϕ_g>, what is the group flux ϕ_1 for the fast energy group (Group 1) and what is the group flux ϕ_2 for the thermal and epithermal energy groups (Group 2)?
The group flux ϕ_g is related to the average flux <ϕ_g> by the equation ϕ_g = <ϕ_g> ⋅ ΔE_g, where ΔE_g is the width of the energy group. The lowest energy group, which contains the thermal and epithermal neutrons, is 100 keV = 1 × 10^5 eV wide, and the fast energy group is 9.9 MeV = 9.9 × 10^6 eV wide. The fast group flux is therefore ϕ_1 = <ϕ_1> ⋅ ΔE_1 = 5 × 10^6 × 9.9 × 10^6 = 4.95 × 10^{13} neutrons/cm²/s, and the thermal and epithermal group flux is therefore ϕ_2 = <ϕ_2> ⋅ ΔE_2 = 2.5 × 10^6 × 1 × 10^5 = 2.5 × 10^{11} neutrons/cm²/s. Notice that the fast flux is much larger than the thermal flux in this case.