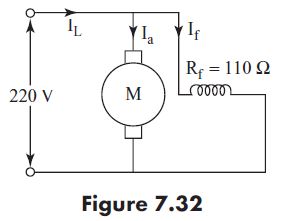
Question 7.8: A 220 V dc shunt motor takes 5 A at no load. The armature re...
A 220 V dc shunt motor takes 5 A at no load. The armature resistance is 0.2 Ω and field resistance is 110 Ω. Calculate the efficiency of the motor when it takes 40 A on full load.
At no-load, I_{L} =5 A
therefore, I_{a} =5-2=3 A
At no-load, when the motor output is zero, the input = V I_{L} (No-load) = 220 × 5 = 1100 W.
The whole of input is lost as I_{a}^{2} R_{a} loss +I_{f}^{2} R_{f} loss + Iron loss + Friction and Windage loss.
\begin{aligned}I_{a}^{2} R_{a} &=5^{2} \times 0.2=5 W \\ I_{f}^{2} R_{f} &=2^{2} \times 110=440 W \end{aligned}Iron, friction, and windage losses = 1100 − 5 − 440 = 655 W
These losses are constant losses and are same at any load. This means, on full load these losses will remain at 655 W.
At full-load, I_{L} =40 A
\begin{aligned} I_{a} &=I_{L}-I_{f}=40-2=38 A \\ I_{a}^{2} R_{a} &=(38)^{2} \times 0.2=289 W \\ I_{f}^{2} R_{f} &=2^{2} \times 110=440 W\end{aligned}Iron, friction, and windages losses = 655 W
Total losses = 289 + 440 + 655 = 1384 W
\begin{aligned} \text {Efficiency} \quad &=\frac{\text { Output }}{\text { Input }}=\frac{\text { Input }-\text { losses }}{\text { Input }} \\&=\frac{(220 \times 40-1384) \times 100}{220 \times 40}=84.3 \text { per cent }\end{aligned}