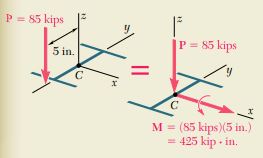
Question 10.SP.7: A steel column having an effective length of 16 ft is loaded...

So that we can select a trial section, we use the allowable-stress method with \sigma_{\text {all }}=22  \mathrm{ksi} and write

\sigma_{\text {all }}=\frac{P}{A}+\frac{M c}{I_{x}}=\frac{P}{A}+\frac{M c}{A r_{x}^{2}}     (1)

From Appendix C we observe for shapes of 8 -in. nominal depth that c \approx 4 in. and r_{x} \approx 3.5  \mathrm{in}. Substituting into Eq. (1), we have

22  \mathrm{ksi}=\frac{85  \mathrm{kips}}{A}+\frac{(425  \mathrm{kip} \cdot \mathrm{in} .)(4  \mathrm{in} .)}{A(3.5  \mathrm{in} .)^{2}} \quad A \approx 10.2 \mathrm{in}^{2}

We select for a first trial shape: \mathrm{W} 8 \times 35.

Trial 1: W8 \times 35. The allowable stresses are

Allowable Bending Stress: (see data ) \quad\left(\sigma_{\text {all }}\right)_{\text {bending }}=22  \mathrm{ksi}

Allowable Concentric Stress: The largest slenderness ratio of the column is L / r_{y}=(192  \mathrm{in}) /.\left(2.03  \mathrm{in}\right..) =94.6. Using Eq. (10.41) with E=29 \times 10^{6}  \mathrm{psi} and \sigma_{Y}=36  \mathrm{ksi}, we find that the slenderness ratio at the junction between the two equations for \sigma_{c r} is L / r=133.7. Thus, we use Eqs. (10.38) and (10.39) and find that \sigma_{c r}=22.5  \mathrm{ksi}. Using Eq. (10.42), the allowable stress is

\left(\sigma_{\text {all }}\right)_{\text {centric }}=22.5 / 1.67=13.46   \mathrm{ksi}

For the W8 \times 35 trial shape, we have

\frac{P}{A}=\frac{85  \mathrm{kips}}{10.3  \mathrm{in}^{2}}=8.25  \mathrm{ksi} \quad \frac{M c}{I}=\frac{M}{S_{x}}=\frac{425   \mathrm{kip} \cdot \mathrm{in} .}{31.2  \mathrm{in}^{3}}=13.62   \mathrm{ksi}

With this data we find that the left-hand member of Eq. (10.60) is

\frac{P / A}{\left(\sigma_{\text {all }}\right)_{\text {centric }}}+\frac{M c / I}{\left(\sigma_{\text {all }}\right)_{\text {bending }}}=\frac{8.25  \mathrm{ksi}}{13.46  \mathrm{ksi}}+\frac{13.62  \mathrm{ksi}}{22  \mathrm{ksi}}=1.232

Since 1.232>1.000, the requirement expressed by the interaction formula is not satisfied; we must select a larger trial shape.

Trial 2: W8 \times 48. Following the procedure used in trial 1, we write

\begin{gathered} \frac{L}{r_{y}}=\frac{192  \mathrm{in} .}{2.08  \mathrm{in} .}=92.3 \quad\left(\sigma_{\text {all }}\right)_{\text {centric }}=13.76  \mathrm{ksi} \\ \frac{P}{A}=\frac{85  \mathrm{kips}}{14.1  \mathrm{in}^{2}}=6.03  \mathrm{ksi} \quad \frac{M c}{I}=\frac{M}{S_{x}}=\frac{425  \mathrm{kip} \cdot \mathrm{in} .}{43.2  \mathrm{in}^{3}}=9.84  \mathrm{ksi} \end{gathered}

Substituting into Eq. (10.60) gives

\frac{P / A}{\left(\sigma_{\text {all }}\right)_{\text {centric }}}+\frac{M c / I}{\left(\sigma_{\text {all }}\right)_{\text {bending }}}=\frac{6.03  \mathrm{ksi}}{13.76  \mathrm{ksi}}+\frac{9.82  \mathrm{ksi}}{22  \mathrm{ksi}}=0.885<1.000

The W8 \times 48 shape is satisfactory but may be unnecessarily large.

Trial 3: W8 \times 40. Following again the same procedure, we find that the interaction formula is not satisfied.

Selection of Shape. The shape to be used is \quad W8 \times 48