Question 7.8: An agitated tank with a standard Rushton impeller is require...
An agitated tank with a standard Rushton impeller is required to disperse gas in a solution of properties similar to those of water. The tank will be 3 m diameter (1 m diameter impeller). A power level of 0.8 kW/m³ is chosen. Assuming fully turbulent conditions and that the presence of the gas does not significantly affect the relation between the Power and Reynolds numbers:
(a) What power will be required by the impeller?
(b) At what speed should the impeller be driven?
(c) If a small pilot scale tank 0.3 m diameter is to be constructed to test the process, at what speed should the impeller be driven?
(a) Assuming that the depth of liquid = tank diameter, then:
volume of liquid = (πD²/4) H
= (π × 3² × 3)/4 = 21.2 m³
With a power input of 0.8 kW/m³, the power required be the impeller is:
P = (0.8 × 21.2) = 17.0 kW
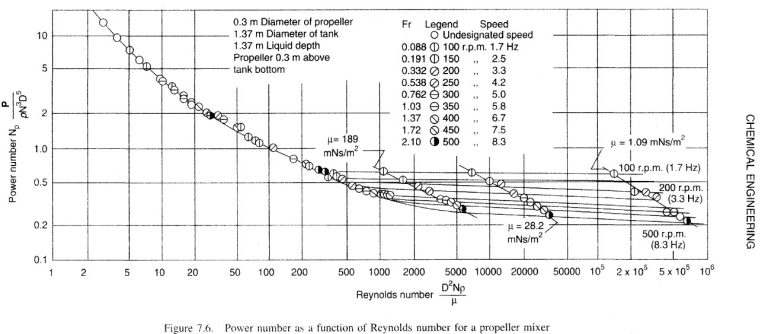
(b) For fully turbulent conditions and μ = 1 mN s/m², and the power number, from Fig. 7.6 is approximately 0.7. On this basis:
P/ρN³D⁵ = 0.7
or: (17.0 × 10³)/(1000N³ × 1⁵) = 0.7
from which: N = 2.90 Hz or 173 rev/ min
(c) For the large tank, from Equation 7.13:
\frac{\boldsymbol{P} }{\rho N_{3}D^{5}} =f\left(\frac{\rho ND^{2}}{\mu } ,\frac{N^{2}D}{g},\frac{D_{T}}{D},\frac{W}{D} ,\frac{H}{D},… \right) (7.13)
P = kN³D⁵
or: (17.0 × 10³) = k × 2.90³ × 15
from which: k = 697
Thus, for the smaller tank, assuming power/unit volume is constant:
volume of fluid = (π/4)0.3² × 0.3 = 0.021 m³
and: power supplied, P = (0.021 × 0.8 × 10³) = 17 W
Thus, for the smaller tank:
17 = 697 N³ × 0.15
and: N = 13.5 Hz or 807 rev/ min .
