Question 8.12: Assume that the series circuit consists of only the resistor...
Assume that the series circuit consists of only the resistor with value R = 2Ω and the capacitor with value C = 0.1 F. Let the initial voltage across the capacitor be v^{-}_{0}= 1 V. Find the current through the circuit and the voltage across the capacitor for t ≥ 0.
Learn more on how we answer questions.
The circuit is shown in Fig. 8.19a in the time-domain. The equivalent frequency-domain circuit is shown in Fig. 8.19b, with a voltage source representing the initial condition. The excitation and the impedance, in the Laplace transform domain, are
\frac{v^{-}_{0}}{s} and R+\left(1/Cs \right).
Therefore,
I (s) =\frac{v^{-}_{0} }{s\left(R+\left(1/Cs \right) \right) }=\frac{v^{-}_{0} }{R} \left(\frac{1}{s+\left(1/RC\right) } \right).
Applying the initial and final value theorems, we get initial and final values of current, respectively, as
\frac{v^{-}_{0}}{R}and 0.
Taking the inverse Laplace transform, we get
i(t) =\frac{v^{-}_{0}}{R}e^{-\frac{t}{RC} }u(t).
The initial and final currents arei^{+}_{0}=v^{-}_{0}/R and i_{\infty =0}. Multiplying current i(t) by the resistance R, we get
v(t)=v^{-}_{0}\left(e^{-\frac{t}{RC} } \right)u(t).
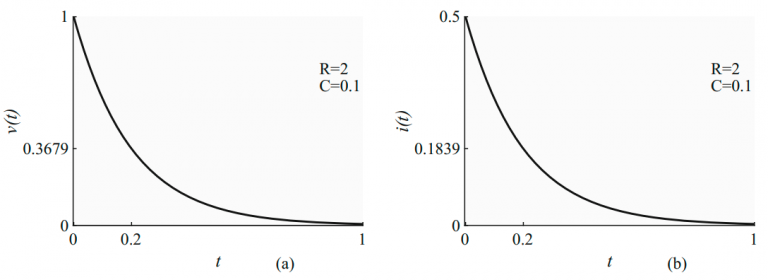
The response of the series RC circuit, for example, with R = 2Ω and C = 0.1 F, to the initial capacitor voltage 1 V is shown in Fig. 8.20. The voltage across the capacitor is shown in (a) and the current in (b).
Using the alternative model shown in Fig. 8.19c, we get
Cv^{-}_{0}=\frac{V(s)}{R}+V(s)C_{s} and V(s)=\frac{v^{-}_{0}}{s+(1/RC)}.
This model is the same as obtained by source transformation. The equivalent source is a current source model of the voltage source.