Question 22.10: Determine the input impedance to the air-core transformer in...
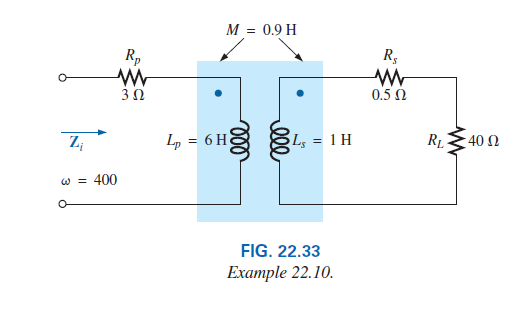
Determine the input impedance to the air-core transformer in Fig. 22.33.
The blue check mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.
Learn more on how we answer questions.
Z _{i}= Z _{p}+\frac{(\omega M)^{2}}{ Z _{s}+ Z _{L}}.
=R_{p}+j X_{L_{p}}+\frac{(\omega M)^{2}}{R_{s}+j X_{L_{s}}+R_{L}}.
=3 \Omega+j 2.4 k \Omega+\frac{((400 rad / s )(0.9 H ))^{2}}{0.5 \Omega+j 400 \Omega+40 \Omega}.
\cong j 2.4 k \Omega+\frac{129.6 \times 10^{3} \Omega}{40.5+j 400}.
=j 2.4 k \Omega+\underbrace{322.4 \Omega \angle-84.22^{\circ}}_{\text {capacitive }}.
=j 2.4 k \Omega+(0.0325 k \Omega-j 0.3208 k \Omega).
=0.0325 k \Omega+j(2.40-0.3208) k \Omega.
\text { and } Z_{i}=R_{i}+j X_{L_{i}}=32.5 \Omega+j 2079 \Omega=2079.25 \Omega \angle 89.10^{\circ}.
Related Answered Questions
Question: 22.4
Verified Answer:
\text { a. } \quad P_{T}=(10)(60 W )+200 W ...
Question: 22.9
Verified Answer:
For each coil, the mutual term is positive, and th...
Question: 22.8
Verified Answer:
\text { Coil 1: } \quad L_{1}\overset{\ove...
Question: 22.7
Verified Answer:
\text { a. } R_{e}=R_{p}+a^{2} R_{s}=1 \Ome...
Question: 22.6
Verified Answer:
a. Ideally, the primary power equals the power del...
Question: 22.5
Verified Answer:
a. The source current:
I_{s}=\frac{E}{R_{T}...
Question: 22.3
Verified Answer:
\text { a. } \frac{I_{p}}{I_{s}}=\frac{N_{...
Question: 22.2
Verified Answer:
\text { a. } E_{p}=4.44 N_{p} f \Phi_{m}[/l...
Question: 22.1
Verified Answer:
\text { a. } M=k \sqrt{L_{p} L_{s}}=0.6 \sq...