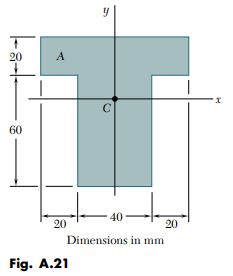
Determine the moment of inertia Ix of the area shown with respect to the centroidal x axis

Question

Determine the moment of inertia [latex]\bar{I}_{x}[/latex] of the area shown with respect to the centroidal [latex]x[/latex] axis (Fig. A.21).

Accepted Answer

Location of Centroid. The centroid [latex]C[/latex] of the area must first be located. However, this has already been done in Example A.02 for the given area. We recall from that example that [latex]C[/latex] is located [latex]46 \mathrm{~mm}[/latex] above the lower edge of the area [latex]A[/latex].

Computation of Moment of Inertia. We divide the area [latex]A[/latex] into the two rectangular areas [latex]A_{1}[/latex] and [latex]A_{2}[/latex] (Fig. A.22), and compute the moment of inertia of each area with respect to the [latex]x[/latex] axis.

Rectangular Area [latex]A_{1}[/latex]. To obtain the moment of inertia [latex]\left(I_{x} ight)_{1}[/latex] of [latex]A_{1}[/latex] with respect to the [latex]x[/latex] axis, we first compute the moment of inertia of [latex]A_{1}[/latex] with respect to its own centroidal axis [latex]x^{\prime}[/latex]. Recalling the formula derived in part [latex]a[/latex] of Example A.04 for the centroidal moment of inertia of a rectangular area, we have

[latex]\left(\bar{I}_{x^{\prime}} ight)_{1}=\frac{1}{12} b h^{3}=\frac{1}{12}(80 \mathrm{~mm})(20 \mathrm{~mm})^{3}=53.3 imes 10^{3} \mathrm{~mm}^{4}[/latex]

Using the parallel-axis theorem, we transfer the moment of inertia of [latex]A_{1}[/latex] from its centroidal axis [latex]x^{\prime}[/latex] to the parallel axis [latex]x[/latex] :

[latex] \begin{aligned} \left(I_{x} ight)_{1}=\left(\bar{I}_{x^{\prime}} ight)_{1}+A_{1} d_{1}^{2}= & 53.3 imes 10^{3}+(80 imes 20)(24)^{2} \ = 975 × 10³ ext{ mm}^4 \end{aligned} [/latex]

Rectangular Area [latex]A_{2}[/latex]. Computing the moment of inertia of [latex]A_{2}[/latex] with respect to its centroidal axis [latex]x^{\prime \prime}[/latex], and using the parallel-axis theorem to transfer it to the [latex]x[/latex] axis, we have

[latex] \begin{aligned} \left(\bar{I}_{x^{\prime \prime}} ight)_{2} & =\frac{1}{12} b h^{3}=\frac{1}{12}(40)(60)^{3}=720 imes 10^{3} \mathrm{~mm}^{4} \ \left(I_{x} ight)_{2} & =\left(\bar{X}_{x^{\prime \prime}} ight)_{2}+A_{2} d_{2}^{2}=720 imes 10^{3}+(40 imes 60)(16)^{2} \ & =1334 imes 10^{3} \mathrm{~mm}^{4} \end{aligned} [/latex]

Entire Area A. Adding the values computed for the moments of inertia of [latex]A_{1}[/latex] and [latex]A_{2}[/latex] with respect to the [latex]x[/latex] axis, we obtain the moment of inertia [latex]\bar{I}_{x}[/latex] of the entire area:

[latex] \begin{aligned} & \bar{I}_{x}=\left(I_{x} ight)_{1}+\left(I_{x} ight)_{2}=975 imes 10^{3}+1334 imes 10^{3} \ & \bar{I}_{x}=2.31 imes 10^{6} \mathrm{~mm}^{4} \end{aligned} [/latex]

Question A.06: Determine the moment of inertia Ix of the area shown with re...

Related Answered Questions

For the circular area of Fig. A.18, determine (a) the polar moment of inertia JO, (b) the ...

Verified Answer:

For the rectangular area of Fig. A.16, determine (a) the moment of inertia Ix of the area ...

Verified Answer:

Referring to the area A of Example A.02, we consider the horizontal x′ axis through its ...

Verified Answer:

Locate the centroid C of the area A shown in Fig. A.10. ...

Verified Answer:

For the triangular area of Fig. A.7, determine (a) the first moment Qx of the area with ...

Verified Answer: