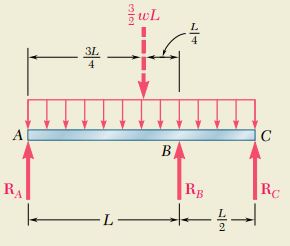
Question 11.SP.7: For the uniform beam and loading shown, determine the reacti...

Castigliano’s Theorem. The beam is indeterminate to the first degree and we choose the reaction \mathbf{R}_{A} as redundant. Using Castigliano’s theorem, we determine the deflection at A due to the combined action of \mathbf{R}_{A} and the distributed load. Since E I is constant, we write

y_{A}=\int \frac{M}{E I}\left(\frac{\partial M}{\partial R_{A}}\right) d x=\frac{1}{E I} \int M \frac{\partial M}{\partial R_{A}} d x     (1)

The integration will be performed separately for portions A B and B C of the beam. Finally, \mathbf{R}_{A} is obtained by setting y_{A} equal to zero.

Free Body: Entire Beam. We express the reactions at B and C in terms of R_{A} and the distributed load

R_{B}=\frac{9}{4} w L-3 R_{A} \quad R_{C}=2 R_{A}-\frac{3}{4} w L    (2)

Portion AB of Beam. Using the free-body diagram shown, we find

M_{1}=R_{A} x-\frac{w x^{2}}{2} \quad \frac{\partial M_{1}}{\partial R_{A}}=x

Substituting into Eq. (1) and integrating from A to B, we have

\frac{1}{E I} \int M_{1} \frac{\partial M}{\partial R_{A}} d x=\frac{1}{E I} \int_{0}^{L}\left(R_{A} x^{2}-\frac{w x^{3}}{2}\right) d x=\frac{1}{E I}\left(\frac{R_{A} L^{3}}{3}-\frac{w L^{4}}{8}\right)    (3)

Portion BC of Beam. We have

M_{2}=\left(2 R_{A}-\frac{3}{4} w L\right) v-\frac{w v^{2}}{2} \quad \frac{\partial M_{2}}{\partial R_{A}}=2 v

Substituting into Eq. (1) and integrating from C, where v=0, to B, where v=\frac{1}{2} L, we have

Reaction at \boldsymbol{A}. Adding the expressions obtained in (3) and (4), we determine y_{A} and set it equal to zero

y_{A}=\frac{1}{E I}\left(\frac{R_{A} L^{3}}{3}-\frac{w L^{4}}{8}\right)+\frac{1}{E I}\left(\frac{R_{A} L^{3}}{6}-\frac{5 w L^{4}}{64}\right)=0

Solving for R_{A}, \quad R_{A}=\frac{13}{32} w L \quad R_{A}=\frac{13}{32} w L \uparrow

Reactions at \boldsymbol{B} and \boldsymbol{C}. Substituting for R_{A} into Eqs. (2), we obtain

R_{B}=\frac{33}{32} w L \uparrow \quad R_{C}=\frac{w L}{16} \uparrow