Question 11.6: Formation of a Neutron Star A star rotates with a period of ...
Formation of a Neutron Star
A star rotates with a period of 30 days about an axis through its center. The period is the time interval required for a point on the star’s equator to make one complete revolution around the axis of rotation. After the star undergoes a supernova explosion, the stellar core, which had a radius of 1.0 × 10^4 km, collapses into a neutron star of radius 10.0 km. Determine the period of rotation of the neutron star.
Learn more on how we answer questions.
Conceptualize The change in the neutron star’s motion is similar to that of the skater described earlier and illustrated in Figure 11.9, but in the reverse direction. As the mass of the star moves closer to the rotation axis, we expect the star to spin faster.
Categorize Let us assume that during the collapse of the stellar core, (1) no external torque acts on it, (2) it remains spherical with the same relative mass distribution, and (3) its mass remains constant. We categorize the star as an isolated system in terms of angular momentum. We do not know the mass distribution of the star, but we have assumed the distribution is symmetric, so the moment of inertia can be expressed as kMR², where k is some numerical constant. (From Table 10.2, for example, we see that k =\frac{2}{5} for a solid sphere and k =\frac{2}{3} for a spherical shell.)
Analyze Let’s use the symbol T for the period, with T_i being the initial period of the star and T_f being the period of the neutron star. The star’s angular speed is given by ω = 2π/T.
From the isolated system model for angular momentum, write Equation 11.21 for the star:
I_i \omega_i=I_f \omega_f=\text{constant} (11.21)
I_i \omega_i=I_f \omega_fUse ω = 2π/T to rewrite this equation in terms of the initial and final periods:
I_i\left(\frac{2 \pi}{T_i}\right)=I_f\left(\frac{2 \pi}{T_f}\right)Substitute the moments of inertia in the preceding equation:
k M R_i{}^2\left(\frac{2 \pi}{T_i}\right)=k M R_f{}^2\left(\frac{2 \pi}{T_f}\right)Solve for the final period of the star:
T_f=\left(\frac{R_f}{R_i}\right)^2 T_iSubstitute numerical values:
T_f=\left(\frac{10.0 km}{1.0 \times 10^4 km}\right)^2(30 \text { days })=3.0 \times 10^{-5} \text { days }=2.6 sFinalize The neutron star does indeed rotate faster after it collapses, as predicted. While it may seem difficult to believe that the core of a star could rotate as fast as once every 2.6 s, this is a relatively slow rotation rate. Some neutron stars rotate with a period of 1–2 milliseconds!
| Table 10.2 Moments of Inertia of Homogeneous Rigid Objects with Different Geometries | ||
| Hoop or thin cylindrical shell I_{CM}= MR^2  |
Long, thin rod with rotation axis through center I_{CM}= \frac{1}{12}ML^2  |
Solid sphere I_{CM}= \frac{2}{5}MR^2 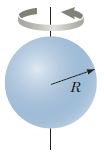 |
| Solid cylinder or disk I_{CM}= \frac{1}{2}MR^2  |
Long, thin rod with rotation axis through end I_{CM}= \frac{1}{3}ML^2  |
|
| Hollow cylinder I_{CM}= \frac{1}{2}M(R_1{}^2+R_2{}^2)  |
Rectangular plate I_{CM}= \frac{1}{12}M(a^2+b^2)  |
Thin spherical shell I_{CM}= \frac{2}{3}MR^2 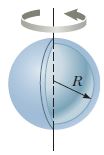 |
