Question 9.6: GROUP AND PHASE VELOCITIES Consider a light wave traveling i...

The phase velocity is given by

v=\frac{c}{n}=\frac{3 \times 10^{8}  m  s ^{-1}}{1.447}=2.073 \times 10^{8}  m  s ^{-1}

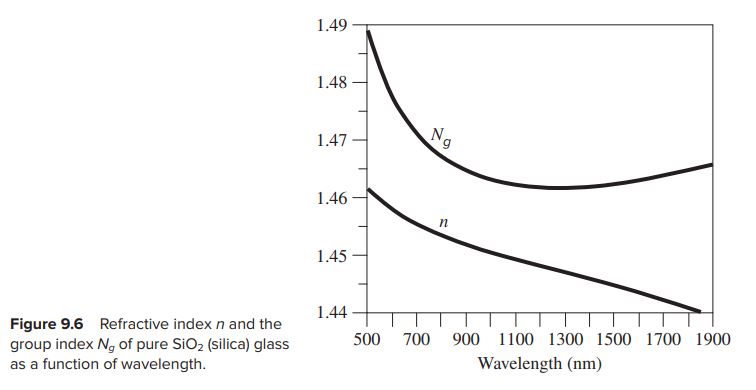
From Figure 9.6, at λ = 1300 nm, N_{g} = 1.462, so

v_{g}=\frac{c}{N_{g}}=\frac{3 \times 10^{8}  m  s ^{-1}}{1.462}=2.052 \times 10^{8}  m  s ^{-1}

The group velocity is ∼0.7 percent smaller than the phase velocity.