Question 13.4.4: Locating Node Points Evaluate the mode shapes and mode frequ...
Locating Node Points
Evaluate the mode shapes and mode frequencies of the vehicle shown in Figure 13.4.2 for the case: k_{1} = 1.6 × 10^{4} N/m, k_{2} = 2.5 × 10^{4} N/m, L_{1} = 1.5 m, L_{2} = 1.1 m, m = 730 kg, and I_{G} = 1350 kg · m² .
Dividing the characteristic equation (13.4.8) by m I_G and using the given values for the constants, we obtain
m I_{G} s^{4} + [m (k_{1}L^{2}_{1} + k_{2}L^{2}_{2}) + I_{G}(k_{1} + k_{2}) ] s^{2} + k_{1} k_{2}(L_{1} + L_{2})^{2} = 0 (13.4.8)
s^{4} + 105.24s^{2} + 2744 = 0The quadratic formula gives the roots s² = −57.61, −47.63. Thus, the four characteristic roots are
s = ±7.59 j, ±6.901 j
These correspond to frequencies of 1.21 Hz and 1.1 Hz.
The mode ratio can be found from equation (1) of Example 13.4.3.
\frac{A_{1}}{A_{2}} = \frac{x}{θ} = \frac{−3500}{730s^{2} + 4.1 × 10^{4}} (1)
For mode 1 (s² = −57.61),
\frac{x}{θ} = \frac{−3500}{730(−57.61) + 4.1 × 10^{4}} = 3.32 m
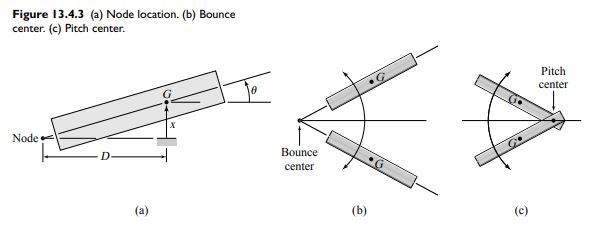
Thus the node is located 3.32 m behind the mass center. Because this node is so far from the mass center, the motion in this mode is predominantly a bounce motion, and this node is called the “bounce center”(see Figure 13.4.3b).
For mode 2 (s² = −47.63),
\frac{x}{θ} = \frac{−3500}{730(−47.63) + 4.1 × 10^{4}} = -0.562 m
This node is located 0.562 m ahead of the mass center (because x/θ < 0). Because this node is close to the mass center, the motion in this mode is predominantly a pitching motion, and this node is called the “pitch center” (see Figure 13.4.3c).
