Question 11.S-P.4: Members of the truss shown consist of sections of aluminum p...
Members of the truss shown consist of sections of aluminum pipe with the cross-sectional areas indicated. Using E = 73 GPa, determine the vertical deflection of point E caused by the load P.
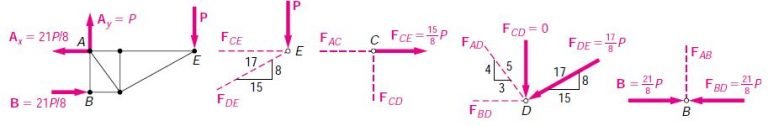
Axial Forces in Truss Members. The reactions are found by using the free-body diagram of the entire truss. We then consider in sequence the equilibrium of joints, E, C, D, and B. At each joint we determine the forces indicated by dashed lines. At joint B, the equation \Sigma F_{x}=0 provides a check of our computations.
\Sigma F_{y}=0: F_{D E}=-\frac{17}{8} P \sum F_{x}=0: F_{A C}=+\frac{15}{8} P \Sigma F_{y}=0: F_{A D}=+\frac{5}{4} P \Sigma F_{y}=0: F_{A B}=0
\Sigma F_{x}=0: F_{C E}=+\frac{15}{8} P \Sigma F_{y}=0: F_{C D}=0 \Sigma F_{x}=0: F_{B D}=-\frac{21}{8} P \Sigma F_{x}=0: (Checks)
Strain Energy. Noting that E is the same for all members, we express the strain energy of the truss as follows
U=\sum \frac{F_{i}^{2} L_{i}}{2 A_{i} E}=\frac{1}{2 E} \sum \frac{F_{i}^{2} L_{i}}{A_{i}} (1)
where F_{i} is the force in a given member as indicated in the following table and where the summation is extended over all members of the truss.
| Member | F _{i} | L_{i}, m | A _{i}, m ^{2} | \frac{ F _{i}^{2} L _{i}}{ A _{i}} |
| AB | 0 | 0.8 | 500 × 10^{-6} | 0 |
| AC | +15P/8 | 0.6 | 500 × 10^{-6} | 4 219P² |
| AD | +5P/4 | 1.0 | 500 × 10^{-6} | 3 125P² |
| BD | -21P/8 | 0.6 | 1000 × 10^{-6} | 4 134P² |
| CD | 0 | 0.8 | 1000 × 10^{-6} | 0 |
| CE | +15P/8 | 1.5 | 500 × 10^{-6} | 10 547P² |
| DE | -17P/8 | 1.7 | 1000 × 10^{-6} | 7 677P² |
Returning to Eq. (1), we have
U=(1 / 2 E)\left(29.7 \times 10^{3} P^{2}\right).
Principle of Work-Energy. We recall that the work done by the load P as it is gradually applied is \frac{1}{2} P y_{E}. Equating the work done by P to the strain energy U and recalling that E = 73 GPa and P = 40 kN, we have
\frac{1}{2} P y_{E}=U \frac{1}{2} P y_{E}=\frac{1}{2 E}\left(29.7 \times 10^{3} P^{2}\right)
y_{E}=\frac{1}{E}\left(29.7 \times 10^{3} P\right)=\frac{\left(29.7 \times 10^{3}\right)\left(40 \times 10^{3}\right)}{73 \times 10^{9}}
y_{E}=16.27 \times 10^{-3} m y_{E}=16.27 mm \downarrow
