Question 7.21: Prove that the ratio of depth to width of the strongest beam...
Prove that the ratio of depth to width of the strongest beam that can be cut from a circular log of diameter d is 1.414. Hence calculate the depth and width of the strongest beam that can be cut of a cylindrical log of wood whose diameter is 300 mm.
Learn more on how we answer questions.
Given :
Dia. of log = d
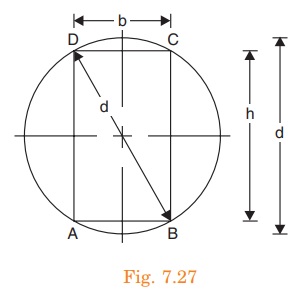
Let ABCD be the strongest rectangular section which can be cut out of the cylindrical log.
Let b = Width of strongest section.
d = Depth of strongest section.
Now section modulus of the rectangular section
Z=\frac{I}{y}=\frac{(\frac{bh^3}{12})}{(\frac{h}{2})}=\frac{bh^2}{6} …(i)
In the above equation, b and h are variable.
From ∆BCD, b² + h² = d²
or h² = d² – b²
Substituting the value of h² in equation (i), we get
Z=\frac{b}{6}[d^2-b^2]=\frac{1}{6}[bd^2-b^3] …(ii)
In the above equation, d is constant and hence only variable is b.
Now for the beam to be strongest, the section modulus should be maximum (or Z should be maximum).
For maximum value of Z,
\frac{dZ}{db}=0
or \frac{d}{db}=[\frac{bd^2-b^3}{6}]=0 \quad \text{ or } \quad \frac{d^2-3b^2}{6}=0
or d² – 3b² = 0 or d² = 3b² …(iii)
But from triangle BCD,
d² = b² + h²
Substituting the value of d² in equation (iii), we get
b² + h² = 3b² or h² = 2b²
or h=\sqrt{2}\times b …(iv)
or \frac{h}{b}=\sqrt{2}=\pmb{1.414.}
Numerical Part
Given, d = 300 mm
But for equation (iii), d² = 3b² or 3b² = d² = 300² = 90000
or b^2=\frac{90000}{3}=30000
∴ b=(30000)^{1/2}=\pmb{173.2 mm.}
From equation (iv),
h=\sqrt{2}\times b=1.414 × 173.2 = \pmb{249.95 mm.}