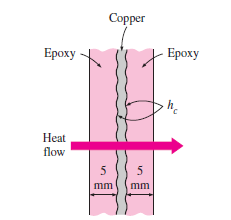
A 1-mm-thick copper plate (k = 386 W/m · °C) is sandwiched between two 5-mm-thick epoxy boards (k = 0.26 W/m · °C) that are 15 cm × 20 cm in size. If the thermal contact conductance on both sides of the copper plate is estimated to be 6000 W/m · °C, determine the error involved in the total thermal

Question

A 1-mm-thick copper plate ([latex] k = 386 W / m \cdot{ }^{\circ} C[/latex]) is sandwiched between two 5-mm-thick epoxy boards ([latex] k = 0.26 W / m \cdot{ }^{\circ} C[/latex]) that are [latex]15 cm imes 20 cm[/latex] in size. If the thermal contact conductance on both sides of the copper plate is estimated to be [latex]6000 W / m \cdot{ }^{\circ} C[/latex], determine the error involved in the total thermal resistance of the plate if the thermal contact conductances are ignored.

Accepted Answer

A thin copper plate is sandwiched between two epoxy boards. The error involved in the total thermal resistance of the plate if the thermal contact conductances are ignored is to be determined.

Assumptions 1 Steady operating conditions exist. 2 Heat transfer is one-dimensional since the plate is large. 3 Thermal conductivities are constant.

Properties The thermal conductivities are given to be [latex] k=386 W / m \cdot{ }^{\circ} C[/latex] for copper plates and [latex] k=0.26 W / m \cdot{ }^{\circ} C[/latex] for epoxy boards. The contact conductance at the interface of copper-epoxy layers is given to be [latex] h_{ c }=6000 W / m ^{2} \cdot{ }^{\circ} C[/latex].

Analysis The thermal resistances of different layers for unit surface area of [latex]1 m ^{2}[/latex] are

[latex] R_{ ext {contact }}=\frac{1}{h_{ c } A_{c}}=\frac{1}{\left(6000 W / m ^{2} \cdot{ }^{\circ} C ight)\left(1 m ^{2} ight)}=0.00017^{\circ} C / W[/latex]

[latex] R_{ ext {plate }}=\frac{L}{k A}=\frac{0.001 m }{\left(386 W / m \cdot{ }^{\circ} C ight)\left(1 m ^{2} ight)}=2.6 imes 10^{-6}{ }^{\circ} C / W[/latex]

[latex] R_{ ext {epoxy }}=\frac{L}{k A}=\frac{0.005 m }{\left(0.26 W / m \cdot{ }^{\circ} C ight)\left(1 m ^{2} ight)}=0.01923^{\circ} C / W[/latex]

The total thermal resistance is

[latex]R_{ ext {total }}=2 R_{ ext {contact }}+R_{ ext {plate }}+2 R_{ ext {epoxy }}[/latex]

[latex] = 2 imes 0.00017+2.6 imes 10^{-6}+2 imes 0.01923 = 0.03914^{\circ} C / W[/latex]

Then the percent error involved in the total thermal resistance of the plate if the thermal contact resistances are ignored is determined to be

[latex]\% ext { Error }=\frac{2 R_{ ext {contact }}}{R_{ ext {total }}} imes 100=\frac{2 imes 0.00017}{0.03914} imes 100= 0 . 8 7 \%[/latex]

which is negligible.

Question 3.48: A 1-mm-thick copper plate (k = 386 W/m · °C) is sandwiched b...

Final Answer

Related Answered Questions