Question 6.1: A column has a solid circular cross section, 1.25 inches in ...

Objective       Specify a safe loading for the column.
Given             Solid circular cross section: diameter = d = 1.25 in; length = L = 4.50 ft.  Both ends of the column are pinned.
Material: AISI 1020 cold-drawn steel.
Analysis          Use the procedure in Figure 6-4.

Results         Step 1. For the pinned-end column, the end-fixity factor is K = 1.0. The effective length equals the actual length; KL = 4.50 ft = 54.0 in.
Step 2. From Appendix 1, for a solid round section,

APPENDIX 1       PROPERTIES OF AREAS
	A = \pi D^4/4              r= D/4 I = \pi D^4/64           J = \pi D^4/32 S = \pi D^3/32                Z_p =\pi D^3/16
	A = \pi (D^2 – d^2)/4      r = \sqrt{D^2 + d^2} /4 I = \pi (D^4 – d^4)/64        J = \pi (D^4 – d^4)/32 S = \pi (D^4 – d^4)/32D          Z_p =\pi (D^4 – d^4)/16D
	A = S^2           r = s/\sqrt{12} I= 5^4/12 S =  5^3/6
	A = BH  r_x = H/\sqrt{12} I_x = BH^3/12         r_y = B/\sqrt{12} S_x = BH^2/6
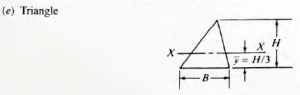	A = BH /2           r = H/\sqrt{18} I = BH^3/36 S = BH^2/24
	A =\pi D^2/8          r= 0.132D I = 0.007D^4 S = 0.024D^3
	A = 0.866D^2         r = 0.264D I = 0.06D^4 S= 0.12D^3
A = area I = moment of inertia S = section modulus r = radius of gyration = \sqrt{I/A} J = polar moment of inertia Z_p = polar section modulus

r = D/4 = 1.25/4 = 0.3125 in

Step 3. Compute the slenderness ratio:

\frac{KL}{r} =\frac{1.0(54)}{0.3125}= 173

Step 4. Compute the column constant from Equation (6-4). For AISI 1020 cold-drawn steel, the yield strength is 51 000 psi. and the modulus of elasticity is 30 ×10^6 psi. Then

C_c=\sqrt{\frac{2\pi ^2E}{s_y}} =\sqrt{\frac{2\pi ^2(30\times 10^6)}{51000}} = 108

Step 5. Because KL/r is greater than C_c, the column is long, and Euler’s formula should be used. The area is

A=\frac{\pi D^2}{4} =\frac{\pi (1.25)^2}{4}=1.23 \ in^2

Then the critical load is

P_{cr}=\frac{\pi ^2EA}{(KL/r)^2}=\frac{\pi ^2(30\times 10^6) (1.23)}{(173)^2}=12 200 lb

At this load, the column should just begin to buckle. A safe load would be a reduced value, found by applying the design factor to the critical load. Let’s use N= 3 to compute the allowable load, P_a = (12 200)/3=4067 lb

Comment       The safe load on the column is 4067 lb.