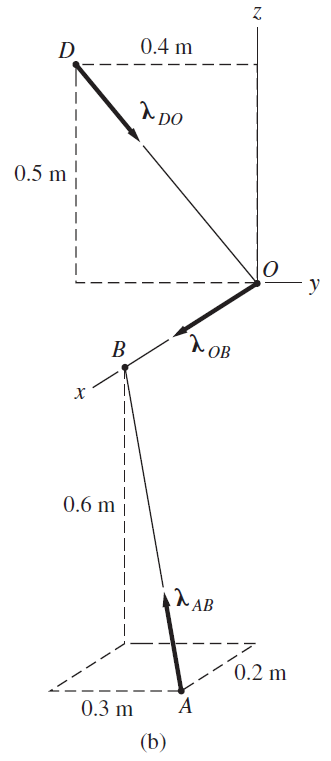
A section of a piping system is acted on by the three couples shown in Fig. (a).Determine the magnitude of the resultant couple-vector C^{R} and its direction cosines, given that the magnitudes of the applied couples are C_{1} =50 N · m, C_{2} =90 N · m, and C_{3} =140 N · m.
Question 2.9: A section of a piping system is acted on by the three couple...

The Blue Check Mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.
Learn more on how we answer questions.
Applying the right-hand rule to each of the three couples in Fig. (a), we see that the corresponding couple-vectors will be directed as follows: C_{1}, from point D toward point O; C_{2}, from point O toward point B; and C_{3}, from point A toward point B. Because these couple-vectors do not have the same directions, the most practical method of determining their resultant is to use the vector equation
C^{R} =C_{1} + C_{2} + C_{3}Using the three unit vectors shown in Fig. (b), the couple-vectors C_{1}, C_{2}, and C_{3} can be written as
C_{1}=C_{1}\lambda _{DO}=50\frac{\overrightarrow{DO} }{\left|\overrightarrow{DO} \right| } =50(\frac{0.4j − 0.5k}{0.6403} )=31.24j − 39.04k N · m C_{2}=C_{2}\lambda _{OB}=90i N.m C_{3}=C_{3}\lambda _{AB}=140\frac{\overrightarrow{AB} }{\left|\overrightarrow{AB} \right| } =140(\frac{−0.2i − 0.3j + 0.6k}{0.7000} )=−40i − 60j + 120k N · mAdding these three couple-vectors gives
C^{R}=50i − 28.76j + 80.96k N · mThe magnitude of C_{R} is
C^{R}=\sqrt{(50)^{2}+(-28.76)^{2}+(80.96)^{2}}=99.41 N.mand the direction cosines of C^{R} are the components of the unit vector λ directed along C^{R}:
\lambda _{x }=\frac{50}{99.41}=0.503 \lambda _{y}=-\frac{28.76}{99.41}=−0.289 \lambda _{z}=\frac{80.96}{99.41}=0.814
The resultant couple-vector is shown in Fig. (c). Although C^{R} is shown at point O, it must be remembered that couples are free vectors, so that C^{R} could be shown acting anywhere.The couple-vector C^{R} can be represented as two equal and opposite parallel forces. However, because the two forces will lie in a plane perpendicular to the couple-vector, in this case a skewed plane, this representation is inconvenient here.In general, given two forces that form a couple, the corresponding couplevector is easily determined (e.g., by summing the moments of the two forces about any point). However, given a couple-vector, it is not always convenient (or even desirable) to determine two equivalent forces.

Related Answered Questions
Moving the given force F from point B to point A r...
Part 1
First we move the 150-kN force to point B, ...
Part 1
The magnitudes (Fd) and senses of the coupl...
Part 1
One method for determining the couple-vecto...
Part 1
Referring to Fig. (a), we see that it is no...
We start by computing the rectangular components o...
The force F and point A lie in the xy-plane. Probl...
Part 1
The moment of a force about point C can be ...
Part 1
The forces are concurrent at point A and th...
Because the three forces are concurrent at point A...