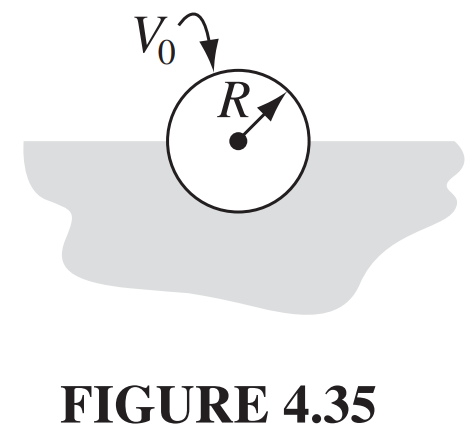
conducting sphere at potential V_{0} is half embedded in linear dielectric material of susceptibility \chi_{e}, which occupies the region z < 0 (Fig. 4.35). Claim: the potential everywhere is exactly the same as it would have been in the absence of the dielectric! Check this claim, as follows:
(a) Write down the formula for the proposed potential V (r), in terms of V_{0}, R, and r. Use it to determine the field, the polarization, the bound charge, and the free charge distribution on the sphere.
(b) Show that the resulting charge configuration would indeed produce the potential V (r).
(c) Appeal to the uniqueness theorem in Prob. 4.38 to complete the argument.
(d) Could you solve the configurations in Fig. 4.36 with the same potential? If not, explain why