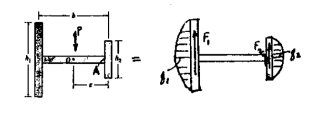
Determine the location e of the shear center, point O, for the thin-walled member having the cross section shown. The member segments have the same thickness t.
Question 7.64: Determine the location e of the shear center, point O, for ...

The Blue Check Mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.
Learn more on how we answer questions.
Summing moments about A ,
e P=b F_{1} \text{(1)}
I=\frac{1}{12}(t)\left(h_{1}\right)^{3}+\frac{1}{12}(t)\left(h_{2}\right)^{3}=\frac{1}{12} t\left(h_{1}^{3}+h_{2}^{3}\right)
q_{1}=\frac{P\left(h_{1} / 2\right)(t)\left(h_{1} / 4\right)}{I}=\frac{P h_{1}^{2} t}{8 I}
F_{1}=\frac{2}{3} q_{1}\left(h_{1}\right)=\frac{P h_{1}^{3} t}{12 I}
From Eq. (1),
e=\frac{b}{P}\left(\frac{P h_{1}^{3} t}{12 I}\right)= \frac{h_{1}^{3} b}{\left(h_{1}^{3}+h_{2}^{3}\right)}
= \frac{b}{1+\left(h_{2}/h_{1}\right)^{3}}
Related Answered Questions
\sigma_{A}=0=\sigma_{a}-\sigma_{b} ...
\bar{y} =\frac{(0.02)(0.2)(0.04)+(0.14)(0.2...
Section Properties:
\bar{y} =\frac{\Sigma \...
\bar{y}=\frac{(0.375)(4)(0.75)+(3.75)(6)(0....
\bar{y}=\frac{(0.375)(4)(0.75)+(3.75)(6)(0....
\bar{y}=\frac{\sum{ \bar{y} A}}{\sum{A}}=\f...
\bar{y}=\frac{\Sigma \bar{y} A}{\Sigma A}=\...
Shear Center. Referring to Fig.a and summing momen...