Determine the rotation, that is, the slope, of the beam ABC shown in Fig. 4.14(a) at A.

Repeat using the Symbolic Math Toolbox in MATLAB.

Question

Determine the rotation, that is, the slope, of the beam ABC shown in Fig. 4.14(a) at A.

Accepted Answer

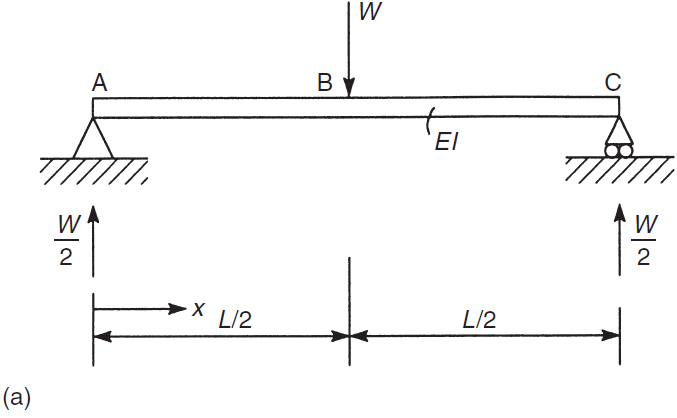
The actual rotation of the beam at A produced by the actual concentrated load, W, is [latex] heta_{ A }[/latex]. Let us suppose that a virtual unit moment is applied at A before the actual rotation takes place, as shown in Fig. 4.14(b). The virtual unit moment induces virtual support reactions of [latex]R_{v, A }(=1 / L)[/latex] acting downward and [latex]R_{v, C }(=1 / L)[/latex] acting upward. The actual internal bending moments are

[latex]M_{ A }=+\frac{W}{2} x, \quad 0 \leq x \leq L / 2[/latex]

[latex]M_{ A }=+\frac{W}{2}(L-x), \quad L / 2 \leq x \leq L[/latex]

The internal virtual bending moment is

[latex]M_{v}=1-\frac{1}{L} x, \quad 0 \leq x \leq L[/latex]

The external virtual work done is [latex]1 heta_{ A }[/latex] (the virtual support reactions do no work, as there is no vertical displacement of the beam at the supports), and the internal virtual work done is given by Eq. (4.21). Hence,

[latex]W_{i, M}=\sum \int_{L} \frac{M_{ A } M_{v}}{E I} d x[/latex] (4.21)

[latex]1 heta_{ A }=\frac{1}{E I}\left[\int_{0}^{L / 2} \frac{W}{2} x\left(1-\frac{x}{L} ight) d x+\int_{L / 2}^{L} \frac{W}{2}(L-x)\left(1-\frac{x}{L} ight) d x ight][/latex] (i)

Simplifying Eq. (i), we have

[latex] heta_{ A }=\frac{W}{2 E I L}\left[\int_{0}^{L / 2}\left(L x-x^{2} ight) d x+\int_{L / 2}^{L}(L-x)^{2} d x ight][/latex] (ii)

Hence,

[latex] heta_{ A }=\frac{W}{2 E I L}\left\{\left[L \frac{x^{2}}{2}-\frac{x^{3}}{3} ight]_{0}^{L / 2}-\frac{1}{3}\left[(L-x)^{3} ight]_{L / 2}^{L} ight\}[/latex]

from which

[latex] heta_{ A }=\frac{W L^{2}}{16 E I}[/latex]

Calculation of the slope of the beam shown in Fig. 4.14(a) at A is obtained through the following MATLAB file:

Question 4.8: Determine the rotation, that is, the slope, of the beam ABC ...

Related Answered Questions