Evaluate the pressure difference between points A and B in Fig. 8.11. Assume aortic values such that v at A is 0.15 m/s, that the diameter at A is 0.03 m, and that the diameter at B is 0.01 m. Assume that ρ=1,060 kg/m^3.

Question

Evaluate the pressure difference between points A and B in Fig. 8.11. Assume aortic values such that [latex]\overline{ u }[/latex] at A is 0.15 m/s, that the diameter at A is 0.03 m, and that the diameter at B is 0.01 m. Assume that [latex] ho =1,060 kg/m^{3}.[/latex]

Accepted Answer

Although Bernoulli should not be used across a sudden expansion, it can be used along a central streamline between sections at A and B. Bernoulli becomes

[latex] \frac{p_{1}}{ ho }+\frac{1}{2} u ^{2}_{1}=\frac{p_{2}}{ ho }+\frac{1}{2} u ^{2}_{2},[/latex]

where [latex] u _{1}A_{1}= u _{2}A_{2}.[/latex] Hence, the pressure difference is

[latex] p_{1}-p_{2}=\frac{1}{2} ho ( u ^{2}_{2}- u ^{2}_{1} )=\frac{1}{2} ho u ^{2}_{1}\left[\left(\frac{A_{1}}{A_{2}} ight)^{2}-1 ight] =\frac{1}{2} ho u ^{2}_{1}\left[\left(\frac{\pi d^{2}_{1}}{\pi d^{2}_{2}} ight)^{2}-1 ight],[/latex]
or
[latex] p_{1}-p_{2}=\frac{1}{2}\left(1060\frac{kg}{m^{3}} ight)\left(0.15\frac{m}{s} ight)^{2}\left[\left(\frac{0.03m}{0.01m} ight)^{4} -1 ight][/latex]

[latex] =954\frac{kg}{ms^{2}}=954\left(kg\frac{m}{s^{2}} ight)/m^{2}=954Pa,[/latex]

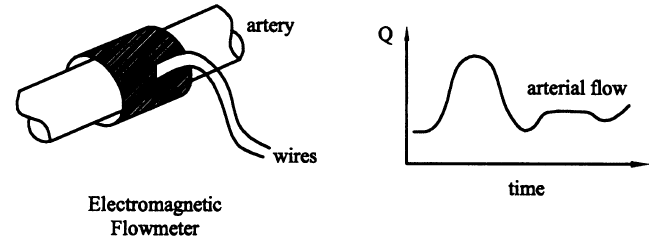
Fundamental Balance Relations FIGURE 8.14 Schema of the time-varying volumetric flow rate Q measured in vivo using an electromagnetic flowmeter. where 7.5 mmHg=1 kPa; hence, [latex]p_{1}-p_{2}=7.155[/latex] mmHg. Note that pressures can be measured chronically in animals using indwelling caheters whereas flows are often measured with implanted flowmeters (e.g., Fig. 8.14).

Question 8.7: Evaluate the pressure difference between points A and B in F...

Related Answered Questions