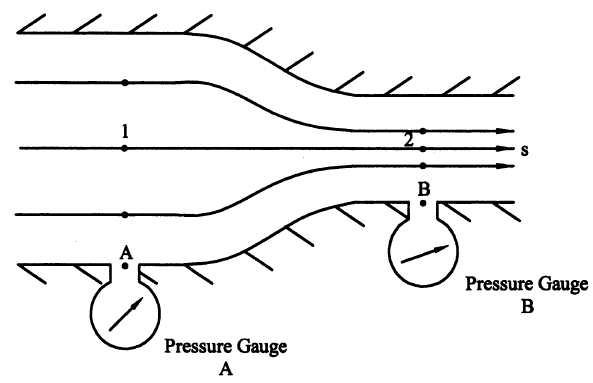
Note that Bernoulli and mass balance provide two equations: \frac{p_{1}}{\rho }=gz_{1}+\frac{1}{2}\nu ^{2}_{1}=\frac{p_{2}}{\rho }+gz_{2}+\frac{1}{2}\nu ^{2}_{2}, \nu _{1}A_{1}=\nu _{2}A_{2}, which can be used to solve for the two velocities, \nu_{1} and \nu_{2}, along a straight horizontal streamline s in a steady, converging, ideal flow, with A_{1} and A_{2} known. To do so, however, we must independently compute or measure the pressures p_{1} and p_{2}. Assuming a negligible gravitational field, determine if the pressure gauges in Fig. 8.9 can be used to determine the pressures along the center streamline.
Question 8.5: Note that Bernoulli and mass balance provide two equations:p...
The Blue Check Mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.
Learn more on how we answer questions.
Because we do not know \nu as a function of (x, y, z) or (r, \theta , z), we cannot determine if Bernoulli holds across the streamline (i.e., if \triangledown \times \nu =0, then Bernoulli may hold for any two points). Hence, let us recall the original Euler equations for a steady ideal flow:
-\frac{\partial p}{\partial s}-\rho g\frac{\partial z}{\partial s}=\rho \nu _{s}\frac{\partial \nu _{s}}{\partial s}, -\frac{\partial p}{\partial n}-\rho g\frac{\partial z}{\partial n}=-\frac{\rho\nu ^{2}_{s} }{R}from Eqs. (8.58) and (8.64). In particular, from the n-direction equation with g\sim0,
-\frac{\partial P}{\partial s}-\rho g\frac{\partial z}{\partial n} =\rho \left(\frac{\partial \nu _{s}}{\partial t}+\nu _{s}\frac{\partial \nu _{s}}{\partial s} \right). (8.58)
-\frac{\partial P}{\partial n}-\rho g\frac{\partial z}{\partial n}=\rho \left(-\frac{\nu ^{2}_{s} }{R} \right). (8.64)
\frac{\partial p}{\partial n}=\frac{\rho\nu ^{2}_{s} }{R},
FIGURE 8.9 A simple internal flow that converges from a larger to a smaller diameter tube. Assume that the pressure gauges are connected flush to the wall of the tubing and that they are filled with an incompressible fluid. where R is the radius of curvature of the streamline. Noting that R\rightarrow \infty for the locally parallel horizontal streamlines within the regions associated with gauges A and B, then at each gauge, \partial p/\partial n=0, which states that p does not vary in the normal direction when the streamlines are locally parallel. Hence, the pressure measured by these gauges, at the wall, equals the pressures at 1 and 2, and Bernoulli and mass balance can determine \nu _{1} and \nu _{2} in terms of measured p_{1}, p_{2}, A_{1} and A_{2}.
Related Answered Questions
For flow along a streamline between points 2 and 4...
\triangledown \cdot \nu =\frac{\partial}{\p...
Assuming that we know the cross-sectional area wit...
Given:
z_{1}=0
A_{1}=\pi...