The effective thermal diffusivity \left\langle \alpha \right\rangle of soil is rather low, under both dry and wet conditions. Because of this, the seasonal variations of the earth’s surface temperature do not penetrate very far from the surface. The seasonal variation of surface temperature for a location is given in Figure (a). The data is the low and high monthly averaged surface temperature. The results are for 24 months and show a nearly periodic time variation. Assume that the difference in extremes in the average earth surface temperature, T_{h} − T_{c}, occurs instantly. This is rendered in Figure (b).
(a) Estimate how far this change penetrates after six months.
(b) Estimate the speed of the penetration u_{F} after three months, i.e., t = \tau /2 , where \tau is the period.
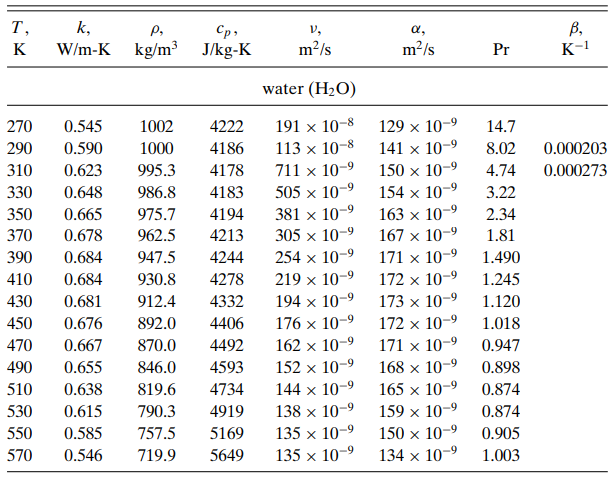
Use the correlation (3.28) for the effective thermal conductivity \left\langle k \right\rangle of a packed bed of particles. For k_{s} use sand that has a high concentration of silicon dioxide (i.e., silica). The properties of silica are listed in Table and we have \rho _{s} = 2,200 kg/m^{3}, c_{p,s} = 745 J/kg-^{\circ }C, and k_{s} = 1.38 W/m-^{\circ }C. For porosity use \epsilon = 0.4 corresponding to random packing of spheres. The properties of air are listed in Table. At T = 300 K, we have \rho_{f} = 1.177 kg/m^{3}, c_{p,f} = 1,005 J/kg-^{\circ}C, and k_{f} = 0.0263 W/m-^{\circ}C. The properties of water are listed in Table. At T = 293 K, we have f = 1,000 kg/m^{3} , c_{p,f} = 4,181 J/kg-^{\circ}C, and k_{f} = 0.597 W/m-^{\circ}C. For the effective volume-specific heat capacity \left\langle \rho c_{p} \right\rangle , use the simple volume averaging given by \left\langle\rho c_{p}\right\rangle _{V}=\left\langle\rho c_{p}\right\rangle \equiv \sum\limits_{i}{\rho _{i}c_{p,i}} =\sum\limits_{i}{\epsilon _{i}\rho _{i}c_{p,i}} .