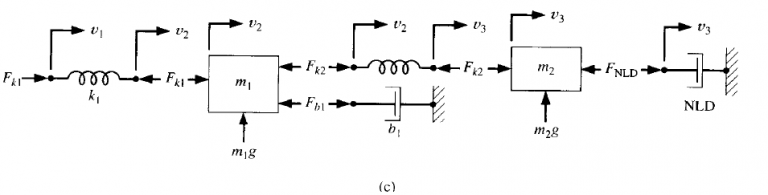
The six-element system shown in Fig. 2.12 is a simplified representation of a vibrating spring–mass assembly (k_1, m_1, b_1) with an attached vibration absorber, subjected to a displacement input x_1, as shown. The object is to develop a mathematical model capable of relating the motions x_2 and x_3 to the input displacement x_1.
Also presented in Fig. 2.12 are diagrams showing the system in an active displaced state and “broken open” for free-body representation.
Question 2.6: The six-element system shown in Fig. 2.12 is a simplified re...


The Blue Check Mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.
Learn more on how we answer questions.
The elemental equation for the spring k_1 in derivative form is
\frac{dF_{k1}}{dt}=k_1(v_1-v_2) (2.35)
Integration of Equation (2.35) with respect to time with x_1 and x_2, both zero in the relaxed state, yields
F_{k1} = k_1(x_1 − x_2). (2.36)
For the mass m_1,
F_{k1} − F_{k2} − F_{b1} = m_1\frac{d^2x_2}{dt^2} . (2.37)
For the damper b_1,
F_{b1} = b_1v_2. (2.38)
For the spring k_2,
F_{k2} = k_2(x_2 − x_3). (2.39)
For the mass m_2,
F_{k2}-F_{NLD}=m_2\frac{d^2x_3}{dt^2} (2.40)
and for the nonlinear damper (NLD),
F_{NLD}=f_{NL}(v_3)=f_{NL}(\frac{dx_3}{dt}) (2.41)
Equations (2.36)–(2.39) may now be combined, yielding
m_1\frac{d^2x_2}{dt^2}+b_1\frac{dx_2}{dt}+(k_1+k_2)x_2=k_1x_1+k_2x_3, (2.42)
and Eqs. (2.39)–(2.41) are combined to yield
m_2\frac{d^2x_3}{dt^2}+f_{NL}\left(\frac{dx_3}{dt}\right) +k_2x_3=k_2x_2. (2.43)
It can be seen that two second-order differential equations are needed to model this fourth-order system (four independent energy-storage elements), one of which is nonlinear. The nonlinear damping term in Eq. (2.43) complicates the algebraic combination of Eqs. (2.42) and (2.43) into a single fourth-order differential equation model. In some cases the NLD characteristic may be linearized, making it possible to combine Eqs. (2.42) and (2.43) into a single fourth-order differential equation for x_2 or x_3. Because this system has four independent energy-storage elements, a set of four state variables is required
for describing the state of this system (e.g., x_2, v_2, x_3, and v_3, or F_{k1}, v_2, F_{k2}, and v_3). The exchange of energy among the input source and the two springs and two masses, together with the energy dissipated by the dampers, would require a very long and complicated verbal description. Thus the mathematical model is a very compact, concise description of the system. Further discussion of the manipulation and solution of this mathematical model is deferred to later chapters.
Related Answered Questions
This problem is solved following the five-step lin...
The complete set of free-body diagrams for this sy...
As an introductory aid in visualizing the action o...
Newton’s second law applied to m – i.e., the eleme...
Newton’s second law applied to the mass m yields
[...
Using Eq. (2.1) –that is, ignoring friction effect...