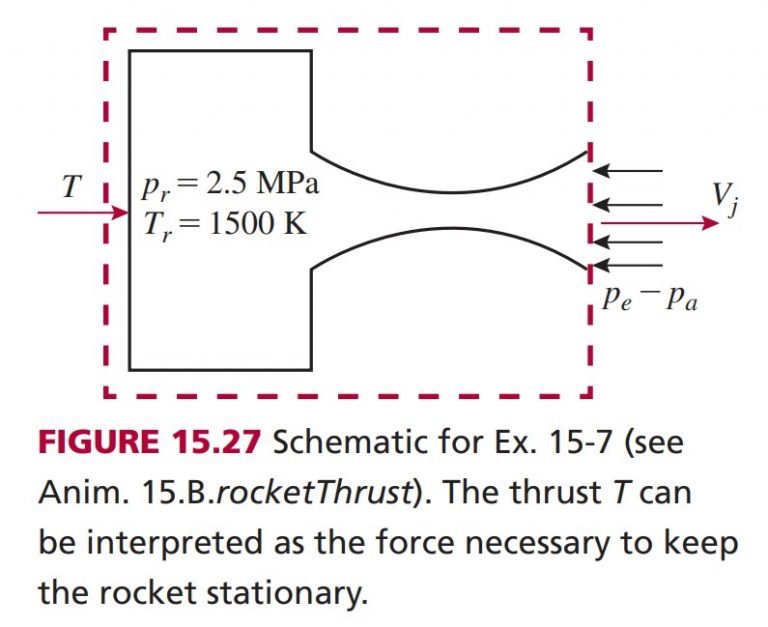
Question 15.7: Thrust Calculation for a Rocket A rocket nozzle with an exit...
Thrust Calculation for a Rocket
A rocket nozzle with an exit area of 0.1 m² is designed to work supersonically with a chamber pressure of 2.5 MPa and a chamber temperature of 1500 K at sea level, where the ambient pressure is 101 kPa. (a) Determine the thrust at sea level. Assume the chamber conditions remain steady. Model the exhaust gas as a perfect gas with k = 1.3 and R = 0.42 kJ/kg ⋅ K. What-if scenario: (b) What would the thrust be if the rocket was in space where the pressure can be assumed to be 0 kPa?
Learn more on how we answer questions.
Calculate the exit velocity and then use Eq. (8.27), derived in Chapter 8, to evaluate the thrust.
Assumption
Isentropic one-dimensional flow of a perfect gas.
Analysis
The thrust equation, Eq. (8.27), can be simplified for the rocket shown in Figure 15.27 by eliminating the inlet transport term.
T=\underbrace{\frac{\dot{m}}{(1000 N / kN )}\left(V_{j}-V_{a}\right)}_{\text {Momentum thrust }}+\underbrace{A_{e}\left(p_{e}-p_{0}\right)-A_{i}\left(p_{i}-p_{0}\right)}_{\text {Pressure thrust }} ; \quad[ kN ] (8.27)
T=\frac{\dot{m}}{(1000 N / kN )}\left(V_{j}- \cancel{V _{a}}^{0}\right)+A_{j}\left(p_{j}-p_{a}\right)=A_{e}\left[\frac{\rho_{e} V_{e}^{2}}{1000}+\left(p_{e}-p_{a}\right)\right]
The pressure thrust in this equation comes from the exit pressure, which is not always equal to the back pressure. The nozzle is designed for sea level; therefore, the exit pressure must be same as the back pressure, 101 kPa, at sea level for isentropic expansion. As a result, p_{e} / p_{t}=101 / 2500=0.0404 . Solving Eqs. (15.25) and (15.24), we obtain M_{e}=2.7 \text { and } T_{e}=715 K .
\frac{T_{t}}{T}=1+\frac{k-1}{2} M^{2} (15.24)
\frac{p_{t}}{p}=\left(\frac{T_{t}}{T}\right)^{k /(k-1)}=\left(1+\frac{k-1}{2} M^{2}\right)^{k /(k-1)} (15.25)
\begin{gathered}V_{e}=M_{e} \sqrt{(1000 N / kN ) k R T_{e}}=2.7 \sqrt{(1000)(1.3)(0.42)(715)}=1690 m / s \\\rho_{e}=\frac{p_{e}}{R T_{e}}=\frac{101}{(0.42)(715)}=0.336 kg / m ^{3}\end{gathered}
At sea level:
T=(0.1)\left[\frac{(0.336) 1690^{2}}{(1000)}+(101-101)\right]=96.0 kN
Because the flow at the exit is supersonic, the exit state is not affected by a reduction in back pressure as the rocket passes through the atmosphere into space. The thrust, however, varies with altitude as p_{a} decreases.
TEST Analysis
Launch the gas dynamics TESTcalc, select Custom working gas, and enter the given values of R and k. Evaluate the chamber state, State-1, from the given properties p1, T1, and Vel1 = 0. Evaluate the isentropic exit state, State-2, from p2 = 101 kPa, p_t2 = p1,T_t2 = T1, and A2 = 0.1m² . In the I/O panel, calculate the thrust by evaluating the expression = mdot2*Vel2/1000 as 96.02 kN.
What-if scenario
The exit velocity remains unchanged in space as the supersonic flow adjusts to the zero back pressure outside the nozzle. However, the pressure component of the thrust increases, producing:
T=(0.1)\left[\frac{(0.336) 1690^{2}}{1000}+(101-0)\right]=106.1 kN
Discussion
The pressure of the exit stream, which remains unchanged at 101 kPa as long as the back pressure is less than 101 kPa, augments the thrust in space by 0.1*(101 – 0) = 10.1 kN. At sea level, the same pressure, 101 kPa, acts all around the system boundary, resulting in no net pressure contribution to the thrust. (see Anim. 15.B.rocketThrust)