Holooly Plus Logo
Holooly Plus Logo

Question 4.9: Design of a Torsion Bar Problem: Determine the best cross-se...

Design of a Torsion Bar

Problem:    Determine the best cross-sectional shape for a hollow torsion bar to be made from a sheet of steel of known dimensions in order to withstand a pure torsional load with minimum angular deflection. Also find the maximum shear stress.

Given:    The applied torque is 10 N-m. The sheet of steel has length ll = 1 m, width ww = 100 mm, and thickness tt = 1 mm. GG = 80.8 GPa.

Assumptions:    Try four different cross-sectional shapes: unformed flat plate, open-circular section, closed-circular section, and closed-square section. The open-circular shape is rolled but is not welded at the seam. The closed shapes’ seams are welded to create a continuous cross section. Assume a mean diameter or mean perimeter consistent with the sheet width.

The blue check mark means that this solution has been answered and checked by an expert. This guarantees that the final answer is accurate.
Learn more on how we answer questions.

See Figure 4-29 and Table 4-3.

1    Equations 4.26 will apply for all sections, provided that we substitute JJ for KK and J/rJ/r for QQ in the case of the closed-circular section.

τmax⁡=TQ \tau_{\max }=\frac{T}{Q}       (4.26a)

θ=TlKG \theta=\frac{T l}{K G}          (4.26b)

2    The unformed flat plate behaves as a solid rectangular section as shown in Figure 4-29a and Table 4-3. It has dimensions aa = w / 2 = 0.05 m and bb = t / 2 = 0.000 5 m:

K=ab3[163−3.36ba⟮1−b412a4⟯]=(0.05)(0.0005)3[5.333−3.360.00050.05⟮1−(0.0005)412(0.05)4⟯]K=3.312E−11 m4=33.123 mm4θ=TlGK=(10)(1)(8.08E10)(3.312E−11)=3.736 rad=214.1∘ \begin{aligned} K &=a b^3\left[\frac{16}{3}-3.36 \frac{b}{a}\left\lgroup1-\frac{b^4}{12 a^4}\right\rgroup \right] \\ &=(0.05)(0.0005)^3\left[5.333-3.36 \frac{0.0005}{0.05}\left\lgroup1-\frac{(0.0005)^4}{12(0.05)^4}\right\rgroup \right] \\ K &=3.312 E-11  m ^4=33.123  mm ^4 \\ \theta &=\frac{T l}{G K}=\frac{(10)(1)}{(8.08 E 10)(3.312 E-11)}=3.736  rad =214.1^{\circ} \end{aligned}                       (a)

This is obviously a rather large angular deflection, indicating that the flat plate has been wound into a corkscrew by this torsional load.

Q=8a2b23a+1.8b=8(0.05)2(0.0005)33(0.05)+1.8(0.0005)Q=3.313E−8 m3=33.13 mm3τ=TQ=103.313E−8=301.8 MPa=43772 psi \begin{aligned} Q &=\frac{8 a^2 b^2}{3 a+1.8 b} \\ &=\frac{8(0.05)^2(0.0005)^3}{3(0.05)+1.8(0.0005)} \\ Q &=3.313 E-8  m ^3=33.13  mm ^3 \\ \tau &=\frac{T}{Q}=\frac{10}{3.313 E-8}=301.8  MPa =43772  psi \end{aligned}         (b)

The maximum shear stress is 300 MPa, which would require a material with a tensile yield strength of over 520 MPa (75 000 psi) in order not to yield and take a set. This requires a high-strength steel. (See Section 5.1 for discussion of this relationship between tensile and shear strength as defined in equation 5.9b. on p. 251.)

Sys=0.577Sy S_{y s}=0.577 S_y     (5.9b)

3    The open-circular shape is now formed into a 3.18-cm diameter tube, but its longitudinal seam is left unwelded and open as shown in Figure 4-29b. The expressions for KK and QQ from Table 4-3 are

K=23πrt3=23π(wπ−t)2t3=13(w−πt)t3=13(0.1−0.001π)0.0013K=3.2286E−11 m4=32.286 mm4θ=TlGK=(10)(1)(8.08E10)(3.2286E−11)=3.833 rad=219.6∘ \begin{aligned} K &=\frac{2}{3} \pi r t^3 \\ &=\frac{2}{3} \pi \frac{\left(\frac{w}{\pi}-t\right)}{2} t^3=\frac{1}{3}(w-\pi t) t^3=\frac{1}{3}(0.1-0.001 \pi) 0.001^3 \\ K &=3.2286 E-11  m ^4=32.286  mm ^4 \\ \theta &=\frac{T l}{G K}=\frac{(10)(1)}{(8.08 E 10)(3.2286 E-11)}=3.833  rad =219.6^{\circ} \end{aligned}        (c)

This is as large an angular deflection as that of the flat plate.

Q=4π2r2t26πr+1.8t=4π2(0.01542)2(0.001)36π(0.01542)+1.8(0.001)Q=3.209E−8 m3=32.09 mm3τ=TQ=103.209E−8=311.6 MPa=45201 psi \begin{aligned} Q &=\frac{4 \pi^2 r^2 t^2}{6 \pi r+1.8 t} \\ &=\frac{4 \pi^2(0.01542)^2(0.001)^3}{6 \pi(0.01542)+1.8(0.001)} \\ Q &=3.209 E-8  m ^3=32.09  mm ^3 \\ \tau &=\frac{T}{Q}=\frac{10}{3.209 E-8}=311.6  MPa =45201  psi \end{aligned}     (d)

The stress and deflection are unacceptable. It is just as bad a design as the flat plate.

4    The closed-square tube is formed by folding the sheet into a square section with side dimension s=a=w/4s = a = w / 4. The seam is welded as shown in Figure 4-29c. From Table 4-3, KK and QQ, and the stress and deflection are now

K=2t2(a−t)42at−2t2=2t2⟮w4−t⟯42w4t−2t2=2(0.001)2⟮0.14−0.001⟯42⟮0.14⟯(0.001)−2(0.001)2K=1.382E−8 m4=13824 mm4θ=TlGK=(10)(1)(8.08E10)(1.382E−8)=0.00895 rad=0.51∘ \begin{array}{l} K=\frac{2 t^2(a-t)^4}{2 a t-2 t^2}=\frac{2 t^2\left\lgroup\frac{w}{4}-t\right\rgroup^4}{2 \frac{w}{4} t-2 t^2}=\frac{2(0.001)^2\left\lgroup\frac{0.1}{4}-0.001\right\rgroup^4}{2\left\lgroup\frac{0.1}{4}\right\rgroup(0.001)-2(0.001)^2} \\ K=1.382 E-8  m ^4=13824  mm ^4 \\ \theta=\frac{T l}{G K}=\frac{(10)(1)}{(8.08 E 10)(1.382 E-8)}=0.00895  rad =0.51^{\circ} \end{array}       (e)

Q=2t(a−t)2=2t⟮w4−t⟯2=2(0.001)⟮0.14−0.001⟯2Q=1.152E−6 m3τ=TQ=101.152E−6=8.7 MPa=1259 psi \begin{array}{l} Q=2 t(a-t)^2=2 t\left\lgroup\frac{w}{4}-t\right\rgroup ^2=2(0.001)\left\lgroup\frac{0.1}{4}-0.001\right\rgroup ^2 \\ Q=1.152 E-6  m ^3 \\ \tau=\frac{T}{Q}=\frac{10}{1.152 E-6}=8.7  MPa =1259  psi \end{array}      (f)

This angular deflection of the square tube is much less than that of either open section, and the maximum shear stress is now much more reasonable.

5    The closed-circular shape is formed into a 3.18-cm outside diameter tube and its longitudinal seam is welded shut as shown in Figure 4-29d. We can either use equations 4.24 and 4.25 (p. 178) or use the general equations 4.26 (p. 178) involving KK and QQ, which are now a function of JJ for this circular shape. For the deflection,

θ=TlJG \theta=\frac{T l}{J G}       (4.24)

J=πd432 J=\frac{\pi d^4}{32}      (4.25a)

J=π(do4−di4)32 J=\frac{\pi\left(d_o^4-d_i^4\right)}{32}      (4.25b)

K=J=π⟮do4−di4⟯32;do=wπ,di=do−2t=π[⟮wπ⟯4−⟮wπ−2t⟯4]32=π[⟮0.1π⟯4−⟮0.1π−2{0.001}⟯4]32K=J=2.304E−8 m4=23041 mm4θ=TlGK=(10)(1)(8.08E10)(2.304E−8)=0.0054 rad=0.31∘ \begin{aligned} K &=J=\frac{\pi\left\lgroup d_o^4-d_i^4\right\rgroup }{32} ; \quad d_o=\frac{w}{\pi}, \quad d_i=d_o-2 t \\ &=\frac{\pi\left[\left\lgroup \frac{w}{\pi} \right\rgroup ^4-\left\lgroup \frac{w}{\pi}-2 t\right\rgroup ^4\right]}{32}=\frac{\pi\left[\left\lgroup \frac{0.1}{\pi}\right\rgroup ^4-\left\lgroup\frac{0.1}{\pi}-2\{0.001\}\right\rgroup ^4\right]}{32} \\ K &=J=2.304 E-8  m ^4=23041  mm ^4 \\ \theta &=\frac{T l}{G K}=\frac{(10)(1)}{(8.08 E 10)(2.304 E-8)}=0.0054  rad =0.31^{\circ} \end{aligned}          (g)

and for the maximum shear stress at the outer surface,

Q=Jr=π⟮do4−di4⟯32ro=π[⟮wπ⟯4−⟮wπ−2t⟯4]32⟮w2π⟯=π[⟮0.1π⟯4−⟮0.1π−2{0.001}⟯4]32⟮0.12π⟯Q=1.448E−6 m3τ=TQ=101.448E−6=6.91 MPa=1002 psi \begin{aligned} Q=\frac{J}{r}=\frac{\pi\left\lgroup d_o^4-d_i^4\right\rgroup }{32 r_o} &=\frac{\pi\left[\left\lgroup\frac{w}{\pi}\right\rgroup ^4-\left\lgroup\frac{w}{\pi}-2 t\right\rgroup ^4\right]}{32\left\lgroup\frac{w}{2 \pi}\right\rgroup }=\frac{\pi\left[\left\lgroup\frac{0.1}{\pi}\right\rgroup ^4-\left\lgroup\frac{0.1}{\pi}-2\{0.001\}\right\rgroup ^4\right]}{32\left\lgroup\frac{0.1}{2 \pi}\right\rgroup } \\ Q &=1.448 E-6  m ^3 \\ \tau &=\frac{T}{Q}=\frac{10}{1.448 E-6}=6.91  MPa =1002  psi \end{aligned}      (h)

6    This closed circular design has the smallest stress and deflection and is clearly the best choice of the four designs presented. The wall thickness could be increased to further reduce stress and deflection if desired. The design needs to be checked for possible torsional buckling as well. The files EX04-09 can be opened in the program of your choice if desired.

Table 4-3 Expressions for K and Q for Some Cross-Section Shapes in Torsion
The Black Dots Indicate Points of Maximum Shear Stress (Source: Ref. 4 with Permission)
Shape K Q
solid square

 K=2.25a4 K=2.25 a^4 Q=a30.6 Q=\frac{a^3}{0.6}
hollow square

 K=2t2(a−t)42at−2t2 K=\frac{2 t^2(a-t)^4}{2 a t-2 t^2} Q=2t(a−t)2 Q=2 t(a-t)^2
inside corners may have higher stress if corner radius is small
solid rectangle

 K=ab3[163−3.36ba⟮1−b412a4⟯] K=a b^3\left[\frac{16}{3}-3.36 \frac{b}{a}\left\lgroup1-\frac{b^4}{12 a^4}\right\rgroup \right] Q=8a2b23a+1.8b Q=\frac{8 a^2 b^2}{3 a+1.8 b}
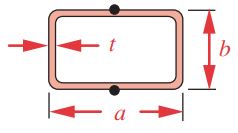
hollow rectangle

 K=2t2(a−t)2(b−t)2at+bt−2t2 K=\frac{2 t^2(a-t)^2(b-t)^2}{a t+b t-2 t^2} Q=2t(a−t)(b−t) Q=2 t(a-t)(b-t)
inside corners may have higher stress if corner radius is small
solid ellipse

 K=πa3b3a2+b2 K=\frac{\pi a^3 b^3}{a^2+b^2} Q=πab22 Q=\frac{\pi a b^2}{2}
hollow ellipse

 K=πa3b3a2+b2[1−⟮1−ta⟯4] K=\frac{\pi a^3 b^3}{a^2+b^2}\left[1-\left\lgroup1-\frac{t}{a}\right\rgroup ^4\right] Q=πab22[1−⟮1−ta⟯4] Q=\frac{\pi a b^2}{2}\left[1-\left\lgroup1-\frac{t}{a}\right\rgroup ^4\right]
open circular tube

 K=23πrt3;t≪r K=\frac{2}{3} \pi r t^3 ; \quad t \ll r Q=4π2r2t26πr+1.8t;t≪r Q=\frac{4 \pi^2 r^2 t^2}{6 \pi r+1.8 t} ; \quad t \ll r
open arbitrary shape

U = length of median line		 K=13Ut3;t≪U K=\frac{1}{3} U t^3 ; \quad t \ll U Q=U2t23U+1.8t;t≪U Q=\frac{U^2 t^2}{3 U+1.8 t} ; \quad t \ll U
t must be much smaller than minimum radius of curvature
F4-29

Related Answered Questions

Question: 4.CS.2B

Crimping-Tool Stress and Deflection Analysis Problem Determine the stresses and deflections at critical points in the crimping tool shown in Figures 3-3 (repeated here) and 4-49. Given The geometry and loading are known from Case Study 2A on p. 84. The thickness of link 1 is 0.313 in, of links 2 ...

Verified Answer:

See Figures 3-3, and 4-49 to 4-51. 1    Link 3 is ...
Question: 4.CS.4B

Bicycle Brake Arm Stress Analysis Problem Determine the stresses at critical points in the bicycle brake arm shown in Figures 3-9 (repeated here) and 4-54. Given The geometry and loading are known from Case Study 4A (p. 94) and are shown in Table 3-5 (p. 97). The cast-aluminum arm is a tee-section ...

Verified Answer:

See Figures 4-54 to 4-56. 1    The brake arm is a ...
Question: 4.CS.3B

Automobile Scissors-Jack Stress and Deflection Analysis Problem Determine the stresses and deflections at critical points in the scissors-jack assembly shown in Figures 3-5 (repeated here) and 4-52. Given The geometry and loading are known from Case Study 3A (p. 88). The design load is 2 000 lb ...

Verified Answer:

See Figures 4-52 to 4-53 and the files CASE3B-1 an...
Question: 4.CS.1B

Bicycle Brake Lever Stress and Deflection Analysis Problem Determine the stresses and deflections at critical points in the brake lever shown in Figures 3-1 (repeated here) and 4-47. Given The geometry and loading are known from Case Study 1A (p. 79). The pivot pin is 8-mm dia. The average human’s ...

Verified Answer:

See Figures 4-47 to 4-48. 1    The 14.3-mm-diamete...
Question: 4.11

Column Design for Concentric Loading Problem: A beachfront house is to be jacked up 10 ft above grade and placed on a set of steel columns. The weight to be supported by each column is estimated to be 200 000 lb. Two designs are to be considered, one using square steel tubes and the other using ...

Verified Answer:

See Table 4-5, parts 1 and 2. 1    This problem, a...
Question: 4.10

Combined Bending and Torsional Stresses Problem Find the most highly stressed locations on the bracket shown in Figure 4-30 and determine the applied and principal stresses at those locations. Given The rod length l = 6 in and arm a = 8 in. The rod outside diameter d = 1.5 in. Load F = 1 000 lb. ...

Verified Answer:

See Figures 4-30 to 4-33. 1    We will limit our i...
Question: 4.8

Finding Reactions of a Statically Indeterminate Beam Using Castigliano’s Method Problem: Find the reaction forces for the indeterminate beam in Figure 4-22e. Given: The load is uniformly distributed over the beam as shown. Length is l. The magnitude of the distributed force is w. Assumptions: ...

Verified Answer:

See Figure 4-22e. 1    Consider the reaction force...
Question: 4.7

Finding Reactions and Deflection of Statically Indeterminate Beams Using Singularity Functions Problem: Determine and plot the loading, shear, moment, slope, and deflection functions for the beam in Figure 4-22d. Find maximum deflection. Given: The load is uniformly distributed over part of the ...

Verified Answer:

See Figures 4-22d (repeated here) and 4-27 (p. 173...
Question: 4.5

Finding Beam Slope and Deflection of a Cantilever Beam Using Singularity Functions Problem: Determine and plot the slope and deflection functions for the beam shown in Figure 4-22b (repeated here). Given: The load is the concentrated force shown. Let beam length l = 10 in, and load location a = 4 ...

Verified Answer:

See Figures 4-22b and 4-24. 1    Write equations f...
Question: 4.6

Finding Beam Slope and Deflection of an Overhung Beam Using Singularity Functions Problem: Determine and plot the slope and deflection functions for an overhung beam with a uniformly distributed load over part of its length and a concentrated force at its end as shown in Figure 4-22c (p. 165). ...

Verified Answer:

See Figures 4-22c (repeated here), 4-25, 4-26. 1  ...