Question 5.9: For the given state of plane stress, construct Mohr’s circle......

We will outline the steps used to construct Mohr’s circle, and make the necessary calculations.
The steps we will follow are

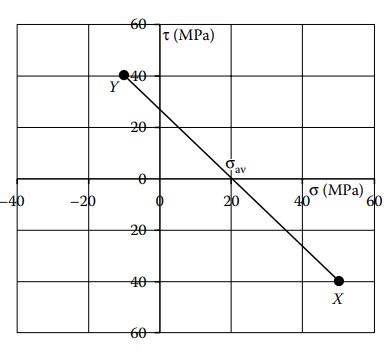
1. Plot point X: (σ_{xx}, \ σ_{xy})
2. Plot point Y: (σ_{yy}, \ σ_{xy})
3. Draw line XY, which passes through the circle center: (σ_{av}, \ 0)
4. Find radius R and draw in the circle

1. Plot point X : (σ_{xx}, \ σ_{xy}): We note straight-off that the shear stress given is positive, according to Figure 4.7, but we are not sure how to plot the point (σ_{xx}, \ σ_{xy}) on Mohr’s circle. Finding σ_{xx} on the σ-axis is straightforward—the normal stress sign convention simply says that tensile stresses are positive and compressive are negative, but does τ_{xy} lie above or below that axis? We know that for Mohr’s circle to work, we must have points X and Y on the opposite sides of the σ-axis, so that their connecting line XY passes through the center of the circle. Our sign convention must ensure this. We, therefore, make use of a system based on the positive x (and y) faces of our unrotated element.

Looking at the positive x face of our initial element (right-hand face), we see that the component of shear stress on this face is tending to rotate the element counterclockwise. This tells us to plot point X below the σ-axis. Our convention is that when this component tends to rotate clockwise, X is above the axis; when counterclockwise, below. (This somewhat awkward rule can be remembered by the equally strange mnemonic: “in the kitchen, the clock is above and the counter is below.”) We formalize this rule in the figure below. Remember that we will apply this sign convention to points X and Y separately—for Mohr’s circle to work, we must have points X and Y on the opposite sides of the σ-axis.

2. Plot point Y: (σ_{yy}, \ σ_{xy}): Following the same reasoning as for point X, we will plot point Y to the left of the τ-axis, as σ_{yy} is compressive, and above the σ-axis, as the shear stress on the positive y (top) face of the element tends to rotate clockwise.
These two points may now be plotted on the στ-axes:

3. Draw line XY: This line passes through the σ (horizontal) axis at the center of the circle:

\left(\sigma_{\mathrm{av}}, 0\right)=\left(\frac{\sigma_{x x}+\sigma_{y y}}{2}, 0\right)=(20  \mathrm{MPa}, 0) .

4. Find the radius R and draw the circle: We may use the geometry of the first three steps, or the formulas derived in the notes, to calculate the radius of the circle. Graphically, we see that R is the hypotenuse of a right triangle whose other legs have length 40 and 50 − 20 = 30. Thus, R = ((40)² + (30)²)^{1/2} = 50 MPa.
Alternatively,

R=\sqrt{\left(\frac{\sigma_{x x}-\sigma_{y y}}{2}\right)^2+\left(\tau_{x y}\right)^2}=50  \mathrm{MPa} .

We can now sketch Mohr’s circle by hand, using a compass, or using a software package. The circle contains all the information we need about all possible axes, and thus all possible stress states, for the given element.

We can find, and label, the principal stresses:

This principal stress state (extreme normal stress, no shear stress) occurs when the axes are rotated by θ_N. (Or when the line XY is rotated around Mohr’s circle by 2θ_N). We can find 2θ_N using a protractor, or we can use our formulas: θ_N = \frac{1}{2} tan^{−1}(2τ_{xy}/(σ_{xx} − σ_{yy})). At this θ_N, we can calculate that the value of \sigma_{x^{\prime} x^{\prime}} (rather than \sigma_{y^{\prime} y^{\prime}}) is 70 MPa, so we draw our properly oriented element which experiences this principal stress state:

Next, we calculate the maximum shear stress and the corresponding normal stress, which we can see from Mohr’s circle is the average normal stress, σ_{av}:

\begin{aligned} \tau_{\max } & =R=50  \mathrm{MPa}, \\ \sigma_{\mathrm{av}} & =20  \mathrm{MPa} \end{aligned}.

From the principal stress state, we can see on Mohr’s circle that it will take 2θ = 90° to obtain this stress state (σ_{av}, \ τ_{max}). We need, then, to rotate our element’s axes 45° counterclockwise past the principal stress orientation. From our initial orientation, this rotation is given by

We can again draw a properly oriented element experiencing the maximum shear stress, having been rotated by 71.6° counterclockwise from its initial orientation:

We obtain the proper sense of the shear stress from Mohr’s circle. By rotating line XY counterclockwise by 2θ_S, we get to a point above the σ-axis. Thus, on the rotated positive x face, we must have a shear stress that tends to rotate the element clockwise.

Finally, we can visualize these rotations on Mohr’s circle:

Note: a positive rotation, that is, a positive value in degrees or radians, is counterclockwise, both in physical space and on Mohr’s circle.