180,000 lb/h of a saturated vapor consisting of 30 mole percent n-butane and 70 mole percent n-pentane are to be condensed at a pressure of 75 psia. The condensing range at feed conditions is from 183.5ºF to 168ºF, and the enthalpy difference between saturated vapor and saturated liquid is

Question

180,000 lb/h of a saturated vapor consisting of 30 mole percent n-butane and 70 mole percent n-pentane are to be condensed at a pressure of 75 psia. The condensing range at feed conditions is from 183.5ºF to 168ºF, and the enthalpy difference between saturated vapor and saturated liquid is 143 Btu/lbm. Cooling water is available at 85ºF and 60 psia. Tubing material is specified to be 90/10 copper–nickel alloy (k = 30 Btu/h . ft .ºF), and a fouling factor of 0.001 h . ft².ºF/Btu is recommended for the cooling water. Maximum pressure drops of 5 psi for the hydrocarbon stream and 10 psi for the coolant are specified. Physical properties of the feed and condensate are given in the table below. The viscosity of the condensate can be estimated using the following equation

$\mu_{L}( CP )=0.00941 \exp \left[1668 / T\left({ }^{\circ} R \right)\right]$

The other condensate properties may be assumed constant at the tabled values. Design a condenser for this service using plain (un-finned) tubes.

Property	Feed	Condensate
$C_{P}\left(\text { Btu/lbm } \cdot{ }^{\circ} F \right)$	0.486	0.61
k (Btu/h . ft .º F)	0.0119	0.057
μ (cp)	0.0085
ρ (lbm/ft³)	0.845	35.5
Pr	0.84

[latex]\mu_{L}( CP )=0.00941 \exp \left[1668 / T\left({ }^{\circ} R ight) ight][/latex]

The other condensate properties may be assumed constant at the tabled values. Design a condenser for this service using plain (un-finned) tubes.

Property	Feed	Condensate
[latex]C_{P}\left( ext { Btu/lbm } \cdot{ }^{\circ} F ight)[/latex]	0.486	0.61
k (Btu/h . ft .º F)	0.0119	0.057
μ (cp)	0.0085
ρ (lbm/ft³)	0.845	35.5
Pr	0.84

Accepted Answer

Make initial specifications.
(i) Fluid placement
A horizontal shell-side condenser will be used. Therefore, the condensing vapor is placed in the shell and cooling water in the tubes.
(ii) Shell and head types
An E-shell unit is specified since it is generally the most economical type of condenser. The difference between the inlet temperatures of the two streams is nearly 100F at design conditions, and could be significantly higher if the temperature of the cooling water decreases during cold weather. Therefore, U-tubes are specified to allow for differential thermal expansion. Thus, an AEU exchanger is specified.
(iii) Tubing
For water service, 3/4-in., 16-BWG tubes are selected with a length of 16 ft.
(iv) Tube layout
Since the shell-side fluid is clean, triangular pitch is specified. A tube pitch of either 15/16 or 1.0 in. can be used. The smaller value is selected because it provides more heat-transfer surface for a given shell size. (See tube-count tables in Appendix C.) (tables C.1, C.2, C.3, C.4, C.5, C.6, C.7 and C.8)

(v) Baffles
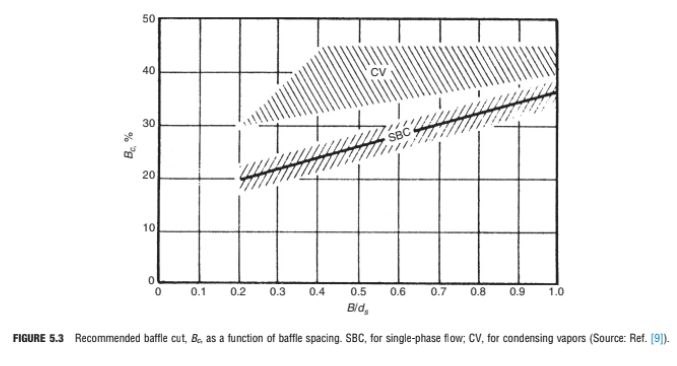
Segmental baffles with a spacing of 0.4 times the shell diameter and a cut of 35% are appropriate for a condensing vapor (see Figure 5.3).
(vi) Sealing strips
The bundle-to-shell clearance in U-tube exchangers is generally small. However, impingement protection is required for a condenser, and some tubes will have to be omitted from the bundle to provide adequate flow area above the impingement plate for the entering vapor. Depending on the size of the resulting gap in the tube bundle, sealing strips may be needed to block the bundle bypass flow.
(vii) Construction materials
The 90/10 cupro–nickel alloy specified for the tubes will provide corrosion resistance and allow a maximum water velocity of 10 ft/s (Table 5.B1). For compatibility, this material is also specified for the tubesheets. Plain carbon steel is adequate for the shell, heads, and all other components.
(b) Energy balances.
The duty is calculated using the given enthalpy difference between saturated vapor and saturated liquid. Condensate subcooling is neglected, as is the effect of pressure drop on saturation temperature:

[latex]q=\dot{m} \Delta H=180,000 imes 143=25 \cdot 74 imes 10^{6} Btu / h[/latex]

Neither the outlet temperature nor the flow rate of the cooling water is specified in this problem. The outlet temperature is frequently limited to 110ºF to 125ºF in order to minimize fouling due to deposition of minerals contained in the water. Economic considerations are also involved, since a higher outlet temperature reduces the amount of cooling water used (which tends to lower the operating cost), while also lowering the mean temperature difference in the exchanger (which tends to increase the capital cost). For the purpose of this example, an outlet temperature of 120ºF will be used, giving an average water temperature of 102.5ºF. The properties of water at this temperature are as follows:

Property	Water at 102.5 ºF
[latex]C_{P}\left( ext { Btu/lbm } \cdot{ }^{\circ} F ight)[/latex]	1
k (Btu/h . ft .ºF)	0.37
μ (cp)	0.72
ρ (lbm/ft³)	0.99
Pr	4.707

The cooling water flow rate is obtained from the energy balance:

[latex]q=25.74 imes 10^{6}=\left(\dot{m} C_{p} \Delta T ight)_{ ext {water }}=\dot{m}_{ ext {water }} imes 1.0 imes 35[/latex]

[latex]\dot{m}_{ ext {water }}=735,429 lbm / h[/latex]

(c) Mean temperature difference.
Since the condensing curve for this system (Figure 11.16) is approximately linear, the mean temperature difference is estimated as follows

[latex]\Delta T_{m} \cong F\left(\Delta T_{\ln } ight)_{c f}[/latex]

[latex]\left(\Delta T_{\ln } ight)_{c f}=\frac{83-63.5}{\ln (83 / 63.5)}=72.8^{\circ} F[/latex]

[latex]R=\frac{T_{a}-T_{b}}{t_{b}-t_{a}}=\frac{183.5-168}{120-85}=0.443[/latex]

[latex]P=\frac{t_{b}-t_{a}}{T_{a}-t_{a}}=\frac{120-85}{183.5-85}=0.355[/latex]

From Figure 3.14, F ≅ 0.98. Therefore,

[latex]\Delta T_{m} \cong 0.98 imes 72.8=71.3^{\circ} F[/latex]

(d) Approximate overall heat-transfer coefficient.
From Table 3.5, for a condenser with low-boiling hydrocarbons on the shell side and cooling water on the tube side, 80 ≤ [latex]U_{D}[/latex] ≤ 200 Btu/h. ft².ºF. Taking the mid-range value gives [latex]U_{D}[/latex] = 140 Btu/h . ft².ºF.

TABLE 3.5 Typical Values of Overall Heat-Transfer Coefficients in Tubular Heat Exchangers. U = Btu/h ft².ºF.

Shell Side	Tube Side	Design U	Includes Total Dirt
Liquid–liquid media
Aroclor 1248	Jet fuels	100–150	0.0015
Cutback asphalt	Water	10–20	0.01
Demineralized water	Water	300–500	0.001
Ethanol amine (MEA or	Water or DEA, or	140–200	0.003
DEA) 10–25% solutions	MEA solutions
Fuel oil	Water	15–25	0.007
Fuel oil	Oil	10–15	0.008
Gasoline	Water	60–100	0.003
Heavy oils	Heavy oils	10–40	0.004
Heavy oils	Water	15–50	0.005
Hydrogen-rich reformer stream	Hydrogen-rich reformer stream	90–120	0.002
Kerosene or gas oil	Water	25–50	0.005
Kerosene or gas oil	Oil	20–35	0.005
Kerosene or jet fuels	Trichloroethylene	40–50	0.0015
Jacket water	Water	230–300	0.002
Lube oil (low viscosity)	Water	25–50	0.002
Lube oil (high viscosity)	Water	40–80	0.003
Lube oil	Oil	11–20	0.006
Naphtha	Water	50–70	0.005
Naphtha	Oil	25–35	0.005
Organic solvents	Water	50–150	0.003
Organic solvents	Brine	35–90	0.003
Organic solvents	Organic solvents	20–60	0.002
Tall oil derivatives, vegetable oil, etc.	Water	20–50	0.004
Water	Caustic soda solutions (10–30%)	100–250	0.003
Water	Water	200–250	0.003
Wax distillate	Water	15–25	0.005
Wax distillate	Oil	13–23	0.005
Condensing vapor-liquid media
Alcohol vapor	Water	100–200	0.002
Asphalt (450ºF)	Dowtherm vapor	40–60	0.006
Dowtherm vapor	Tall oil and derivatives	60–80	0.004
Dowtherm vapor	Dowtherm liquid	80–120	0.0015
Gas-plant tar	Steam	40–50	0.0055
High-boiling hydrocarbons V	Water	20–50	0.003
Low-boiling hydrocarbons A	Water	80–200	0.003
Hydrocarbon vapors (partial condenser)	Oil	25–40	0.004
Organic solvents A	Water	100–200	0.003
Organic solvents high NC, A	Water or brine	20–60	0.003
Organic solvents low NC, V	Water or brine	50–120	0.003
Kerosene	Water	30–65	0.004
Kerosene	Oil	20–30	0.005
Naphtha	Water	50–75	0.005
Naphtha	Oil	20–30	0.005
Stabilizer reflux vapors	Water	80–120	0.003
Steam	Feed water	400–1000	0.0005
Steam	No. 6 fuel oil	15–25	0.0055
Steam	No. 2 fuel oil	60–90	0.0025
Sulfur dioxide	Water	150–200	0.003
Tall-oil derivatives, vegetable oils (vapor)	Water	20–50	0.004
Water	Aromatic vapor-stream	40–80	0.005
	azeotrope
Gas-liquid media
Air, [latex]N_2[/latex], etc. (compressed)	Water or brine	40–80	0.005
Air, [latex]N_2[/latex], etc., A	Water or brine	10–50	0.005
Water or brine	Air, [latex]N_2[/latex] (compressed)	20–40	0.005
Water or brine	Air, [latex]N_2[/latex], etc., A	5–20	0.005
Water	Hydrogen containing natural–gas mixtures	80–125	0.003
Vaporizers
Anhydrous ammonia	Steam condensing	150–300	0.0015
Chlorine	Steam condensing	150–300	0.0015
Chlorine	Light heat-transfer oil	40–60	0.0015
Propane, butane, etc.	Steam condensing	200–300	0.0015
Water	Steam condensing	250–400	0.0015

NC: non-condensable gas present; V: vacuum; A: atmospheric pressure.
Dirt (or fouling factor) units are (h) (ft²) (ºF)/Btu

(e) Heat-transfer area and number of tubes

[latex]A=\frac{q}{U_{D} \Delta T_{m}}=\frac{25.74 imes 10^{6}}{140 imes 71.3}=2579 ft ^{2}[/latex]

[latex]n_{t}=\frac{A}{\pi D_{o} L}=\frac{2579}{\pi(0.75 / 12) imes 16}=821[/latex]

(f) Number of tube passes.

[latex]D_{i}[/latex] = 0.620 in. = 0.0517 ft (Table B.1)

[latex]R e=\frac{4 \dot{m}\left(n_{p} / n_{t} ight)}{\pi D_{i} \mu}=\frac{4 imes 735,429\left(n_{p} / 821 ight)}{\pi imes 0.0517 imes 0.72 imes 2.419}=12,666 n_{p}[/latex]

Therefore, two tube passes will suffice to give fully turbulent flow in the tubes. Checking the fluid velocity,

[latex]V=\frac{\dot{m}\left(n_{p} / n_{t} ight)}{ ho \pi D_{i}^{2} / 4}=\frac{(735,429 / 3600)(2 / 821)}{0.99 imes 62.43 imes \pi(0.0517)^{2} / 4}=3.84 ft / s[/latex]

The velocity is acceptable, and therefore two passes will be used. (Note that four passes could also be used, giving a velocity of about 7.7 ft/s.)

(g) Shell size and tube count.
From Table C.2, the closest count is 846 tubes in a 31-in. shell.
(h) Required overall coefficient.

[latex]U_{r e q}=\frac{q}{n_{ t } \pi D_{o} L \Delta T_{m}}=\frac{25.74 imes 10^{6}}{846 imes \pi imes(0.75 / 12) imes 16 imes 71.3}=136 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(i) Calculate [latex]h_{i}[/latex] assuming [latex]Φ_{i}[/latex] = 1.0.

[latex]R e=\frac{4 \dot{m}\left(n_{p} / n_{t} ight)}{\pi D_{i} \mu}=\frac{4 imes 735,429(2 / 846)}{\pi imes 0.0517 imes 0.72 imes 2.419}=24,584[/latex]

[latex]h_{i}=\left(k / D_{i} ight) imes 0.023 R e^{0.8} \operatorname{Pr}^{1 / 3}\left(\mu / \mu_{w} ight)^{0.14}[/latex]

[latex]=(0.37 / 0.0517) imes 0.023(24,584)^{0.8}(4.707)^{1 / 3} imes 1.0[/latex]

[latex]h_{i}=898 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(j) Calculate [latex]h_{0}[/latex]
The basic Nusselt theory will be used to obtain a conservative estimate for the condensing heat-transfer coefficient. A tube wall temperature of 125º F is assumed to start the calculation. The average film temperature is calculated using the average vapor temperature of 175.75ºF:

[latex]T_{f}=0.75 T_{w}+0.25 T_{V}=0.75 imes 125+0.25 imes 175.75=137.7^{\circ} F[/latex]

The condensate viscosity at this temperature is:

[latex]\mu_{L}=0.00941 \exp [1668 /(137.7+460)]=0.153 cp[/latex]

The modified condensate loading is calculated from Equation (11.41):

[latex]\Gamma^{*}=\frac{W}{L n_{t}^{2 / 3}}=\frac{180,000}{16(846)^{2 / 3}}=125.77 lbm / h \cdot ft[/latex]

The heat-transfer coefficient is given by Equation (11.40):

[latex]h_{o}=1.52\left[\frac{k_{L}^{3} ho_{L}\left( ho_{L}- ho_{V} ight) g}{4 \mu_{L} \Gamma^{*}} ight]^{1 / 3}=1.52\left[\frac{(0.057)^{3} imes 35.5(35.5-0.845) imes 4.17 imes 10^{8}}{4 imes 0.153 imes 2.419 imes 125.77} ight]^{1 / 3}[/latex]

[latex]h_{o}=121 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

The shell-side coefficient is less than [latex]U_{r e q}[/latex], indicating that the condenser is severely under-sized. Therefore, no further calculations will be made with the initial configuration.
The low value of ho suggests that the value of [latex]U_{D}[/latex] is likely to be less than 100 Btu/h . ft².ºF. The number of tubes needed to give [latex]U_{r e q}[/latex] a value of, say, 90 Btu/h h . ft².ºF is:

[latex]\left(n_{t} ight)_{ ext {req }}=846(136 / 90)=1278[/latex]

With this many tubes, four tube passes will be required to keep the water velocity above 3 ft/s. Referring to the tube-count table, a 39-in. shell containing 1336 tubes is selected for the next trial.

Second Trial
(a) Required overall coefficient.

[latex]U_{r e q}=\frac{q}{n_{ t } \pi D_{0} L \Delta T_{m}}=\frac{25.74 imes 10^{6}}{1336 imes \pi(0.75 / 12) imes 16 imes 71.3}=86 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(b) Calculate [latex]h_{i}[/latex] assuming [latex]\phi_{i}[/latex] = 1.0.

[latex]R e=\frac{4 \dot{m}\left(n_{p} / n_{t} ight)}{\pi D_{i} \mu}=\frac{4 imes 735,429(4 / 1336)}{\pi imes 0.0517 imes 0.72 imes 2.419}=31,135[/latex]

[latex]h_{ i }=\left(k / D_{i} ight) imes 0.023 R e^{0.8} \operatorname{Pr}^{1 / 3}\left(\mu / \mu_{w} ight)^{0.14}[/latex]

[latex]=(0.37 / 0.0517) imes 0.023(31,135)^{0.8}(4.707)^{1 / 3} imes 1.0[/latex]

[latex]h_{i}=1085 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(c) Calculate [latex]h_{0}[/latex].
As before, a wall temperature of 125ºF is assumed, giving an average film temperature of 137.7ºF, at which [latex]μ_{L} [/latex] = 0.153 cp.

[latex]\Gamma^{*}=\frac{W}{L n_{t}^{2 / 3}}=\frac{180,000}{16(1336)^{2 / 3}}=92.74 lbm / h \cdot ft[/latex]

[latex]h_{0}=1.52\left[\frac{(0.057)^{3} imes 35.5(35.5-0.845) imes 4.17 imes 10^{8}}{4 imes 0.153 imes 2.419 imes 92.74} ight]^{1 / 3}[/latex]

[latex]h_{0}=134 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(d) Calculate [latex]T_{w} and T_{f}[/latex] .

[latex]T_{w}=\frac{h_{i} t_{a v e}+h_{o}\left(D_{o} / D_{i} ight) T_{a v e}}{h_{i}+h_{o}\left(D_{o} / D_{i} ight)}=\frac{1085 imes 102.5+134(0.75 / 0.62) imes 175.75}{1085+134(0.75 / 0.62)}[/latex]

[latex]T_{w}=112^{\circ}F[/latex]

[latex]T_{f}=0.75 imes 112+0.25 imes 175.75=128^{\circ}F[/latex]

(e) Recalculate [latex]h_{0}[/latex] .
The value of [latex]\mu_{L}[/latex] at 128ºF is:

[latex]\mu_{L}=0.00941 \exp [1668 /(128+460)]=0.161 cp[/latex]

The corresponding value of [latex]h_{0}[/latex] is:

[latex]h_{0}=1.52\left[\frac{(0.057)^{3} imes 35.5(35.5-0.845) imes 4.17 imes 10^{8}}{4 imes 0.161 imes 2.419 imes 92.74} ight]^{1 / 3}=132 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

Since this value is close to the previous one, no further iterations are required.
(f) Viscosity correction factor for cooling water.
Since the tube wall temperature is close to the bulk water temperature, the viscosity correction factor is neglected.
(g) Overall coefficient.
The recommended fouling factor for the cooling water was given in the problem statement as 0.001 h . ft².ºF/Btu. Since the condensing vapor is a very clean stream, a fouling factor of 0.0005 h . ft².ºF/Btu is appropriate. Thus,

[latex]U_{D}=\left[\frac{D_{o}}{h_{i} D_{i}}+\frac{D_{o} \ln \left(D_{o} / D_{i} ight)}{2 k_{ ext {tube }}}+\frac{1}{h_{o}}+\frac{R_{D i} imes D_{o}}{D_{i}}+R_{D o} ight]^{-1}[/latex]

[latex]=\left[\frac{0.75}{1085 imes 0.62}+\frac{(0.75 / 12) \ln (0.75 / 0.62)}{2 imes 30}+\frac{1}{132}+\frac{0.001 imes 0.72}{0.62}+0.0005 ight]^{-1}[/latex]

[latex]U_{D}=94 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

(h) Correction for sensible heat transfer.
The effect of interfacial shear was neglected in calculating [latex]h_{0}[/latex], which will more than offset the effect of sensible heat transfer. Hence, this step could be omitted, but is included here to illustrate the procedure. The rate of sensible heat transfer is estimated using Equation (11.73). For convenience, the heat capacity at vapor inlet conditions is used as an approximation for [latex]\bar{C}_{P, V}[/latex] :

[latex]q_{s e n}=0.5 \bar{C}_{P, V}\left(\dot{m}_{V, ext { in }}+\dot{m}_{V, ho u t} ight)\left(T_{V, ext { in }}-T_{V, ext { out }} ight)[/latex]

[latex]=0.5 imes 0.486 imes 180,000(183.5-168)[/latex]

[latex]q_{ sen }=677,970 Btu / h[/latex]

[latex]q_{ sen } / q_{T o t}=677,970 / 25.74 imes 10^{6} \cong 0.026[/latex]

The Simplified Delaware method will be used to calculate [latex]h_{V}[/latex]. Since the baffle cut is greater than 20%, this method will tend to overestimate[latex]h_{V}[/latex]. However, the safety factor built into the method will help to compensate for this error:

[latex]B=0.4 d_{5}=0.4 imes 39=15.6 ext { in. }[/latex]

[latex]C^{\prime}=P_{T}-D_{0}=15 / 16-0.75=0.1875 in .[/latex]

[latex]a_{5}=\frac{d_{5} C^{\prime} B}{144 P_{T}}=\frac{39 imes 0.1875 imes 15.6}{144 imes 15 / 16}=0.845 ft ^{2}[/latex]

[latex]D_{e}=0.55 / 12=0.04583 ft \quad ext { (from Figure 3.17) }[/latex]

The average vapor flow rate of 90,000 lb/h is used to calculate [latex]h_{V}[/latex]. Physical properties of the vapor are taken at inlet conditions for convenience

[latex]G=\dot{m} / a_{5}=90,000 / 0.845=106,509 lbm / h \cdot ft ^{2}[/latex]

[latex]R e=\frac{D_{e} G}{\mu_{V}}=\frac{0.04583 imes 106,509}{0.0085 imes 2.419}=237,400[/latex]

[latex]j_{H}=0.5\left(1+B / d_{s} ight)\left(0.08 R e^{0.6821}+0.7 R e^{0.1772} ight)[/latex]

[latex]=0.5(1+0.4)\left\{0.08(237,400)^{0.6821}+0.7(237,400)^{0.1772} ight\}[/latex]

[latex]j_{H}=264.3[/latex]

[latex]h_{V}=j_{H}\left(k / D_{e} ight) \operatorname{Pr}^{1 / 3}\left(\mu / \mu_{w} ight)^{0.14}[/latex]

[latex]=264.3(0.0119 / 0.04583)(0.84)^{1 / 3}(1.0)[/latex]

[latex]h_{V}=65 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

The modified overall coefficient is given by Equation (11.72):

[latex]U_{D}^{\prime}=\left[U_{D}^{-1}+\left(q_{\operatorname{sen}} / q_{T o t} ight) h_{V}^{-1} ight]^{-1}[/latex]

[latex]=\left[(94)^{-1}+0.026(65)^{-1} ight]^{-1}[/latex]

[latex]U_{D}^{\prime}=91 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

Since [latex]U_{D}^{\prime} > U_{req}[/latex] , the condenser is thermally acceptable
(i) Tube-side pressure drop

[latex] f=0.4137 R e^{-0.2585}=0.4137(31,135)^{-0.2585}=0.0285[/latex]

[latex]G=\frac{\dot{m}\left(n_{p} / n_{t} ight)}{A_{f}}=\frac{735,429(4 / 1336)}{(\pi / 4)(0.0517)^{2}}=1,048,874 lbm / h \cdot ft ^{2}[/latex]

[latex]\Delta P_{f}=\frac{f n_{p} L G^{2}}{7.50 imes 10^{12} D_{i} s \phi}=\frac{0.0285 imes 4 imes 16(1,048,874)^{2}}{7.50 imes 10^{12} imes 0.0517 imes 0.99 imes 1.0}[/latex]

[latex]\Delta P_{f}=5.23 psi[/latex]

[latex]\Delta P_{ r }=1.334 imes 10^{-13} \alpha_{ r } G^{2} / s[/latex]

From Table 5.1, for turbulent flow in U-tubes:

TABLE 5.1 Number of Velocity Heads Allocated for Minor Losses on Tube Side

Flow Regime	Regular Tubes	U-Tubes
Turbulent	2[latex] n_{p} [/latex]– 1.5	1.6 [latex] n_{p} [/latex] – 1.5
Laminar, Re ≥ 500	3.25 [latex] n_{p} [/latex] – 1.5	2.38 [latex] n_{p} [/latex] – 1.5

[latex]\alpha_{r}=1.6 n_{p}-1.5=1.6 imes 4-1.5=4.9[/latex]

Hence,

[latex]\Delta P_{ r }=1.334 imes 10^{-13} imes 4.9(1,048,874)^{2} / 0.99=0.75 psi[/latex]

Table 5.3 indicates that 10-in. nozzles are appropriate for this unit. Assuming that schedule 40 pipe is used, the Reynolds number for the nozzles is:

TABLE 5.3 Guidelines for Sizing Nozzles

Shell Size, Inches	Nominal Nozzle Diameter, Inches
4–10	2
12–17.25	3
19.25–21.25	4
23–29	6
31–37	8
39–42	10

[latex]R e_{n}=\frac{4 \dot{m}}{\pi D_{i} \mu}=\frac{4 imes 735,429}{\pi(10.02 / 12) imes 0.72 imes 2.419}=643,867[/latex]

[latex]G_{n}=\frac{\dot{m}}{(\pi / 4) D_{i}^{2}}=\frac{735,429}{(\pi / 4)(10.02 / 12)^{2}}=1,343,006 lbm / h \cdot ft ^{2}[/latex]

Since the flow is turbulent, Equation (5.4) is used to estimate the pressure loss in the nozzles:

[latex]\Delta P_{n}=2.0 imes 10^{-13} N_{s} G_{n}^{2} / s=2.0 imes 10^{-13} imes 1(1,343,006)^{2} / 0.99[/latex]

[latex]\Delta P_{n}=0.36 psi[/latex]

The total tube-side pressure drop is:

[latex]\Delta P_{i}=\Delta P_{f}+\Delta P_{f}+\Delta P_{n}=5.23+0.73+0.36 \cong 6.3 psi[/latex]

(j) Shell-side pressure drop.

[latex]\Delta P_f=\bar{\phi }_{VO}^2(\Delta P_f)_{VO}[/latex] (11.69)

Equation (11.69) will be used to estimate the pressure drop, and the Simplified Delaware method will be used to calculate [latex]\left(\Delta P_{f} ight)_{VO}[/latex]. Due to the relatively large baffle cut, the Simplified Delaware method will tend to overestimate the actual pressure drop. (The full Delaware method could be used to obtain a more accurate estimate of the pressure drop, but the additional computational effort is not justified in the present context.) From step (h) we have:

B = 15.6 in

[latex]a_{s}=0.845 ft ^{2}[/latex]

[latex]D_{e}=0.04583 ft[/latex]

[latex]G=\dot{m}_{V} / a_{s}=180,000 / 0.845=213,018 lbm / h \cdot ft ^{2}[/latex]

[latex]R e=\frac{D_{e} G}{\mu_{V}}=\frac{0.04583 imes 213,018}{0.0085 imes 2.419}=474,801[/latex]

The friction factor is calculated using Equations (5.7) and (5.9). Note that since the shell diameter exceeds 23.25 in., [latex]d_{s}[/latex] is set to 23.25 in Equation (5.9):

[latex]f_{1}=\left(0.0076+0.000166 d_{5} ight) R e^{-0.125}[/latex]

[latex]=(0.0076+0.000166 imes 39)(474,801)^{-0.125}[/latex]

[latex]f_{1}=0.00275 ft ^{2} / ext { in. }^{2}[/latex]

[latex]f_{2}=\left(0.0016+5.8 imes 10^{-5} d_{s} ight) R e^{-0.157}[/latex]

[latex]=\left(0.0016+5.8 imes 10^{-5} imes 23.25 ight)(474,801)^{-0.157}[/latex]

[latex]f_{2}=0.000379 ft ^{2} / ext { in. }^{2}[/latex]

[latex]f=144\left\{f_{1}-1.25\left(1-B / d_{s} ight)\left(f_{1}-f_{2} ight) ight\}[/latex]

[latex]=144\{0.00275-1.25(1-0.4)(0.00275-0.000379)\}[/latex]

f = 0.140

The number of baffle spaces is estimated as:

[latex]n_{b}+1=L / B=\frac{16 imes 12}{15.6} \cong 12.3 \Rightarrow 12[/latex]

The pressure drop for the total flow as vapor is calculated from Equation (5.6):

[latex]\left(\Delta P_{f} ight)_{V O}=\frac{f G^{2} d_{s}\left(n_{b}+1 ight)}{7.50 imes 10^{12} D_{e} s \phi}=\frac{0.140(213,018)^{2}(39 / 12)(12)}{7.50 imes 10^{12} imes 0.04583(0.845 / 62.43)(1.0)}[/latex]

[latex]\left(\Delta P_{f} ight)_{V O}=53.3 psi[/latex]

From Equation (11.70),

[latex]\bar{\phi}_{V O}^{2}=0.33+0.22x_e+0.61x_e^2[/latex] [latex](0\leq x_e\leq 0.95)[/latex] (11.70)

[latex]\bar{\phi}_{V O}^{2}=[/latex] 0.33 for a total condenser. Therefore,

[latex]\Delta P_{f}=\bar{\phi}_{V O}^{2}\left(\Delta P_{f} ight)_{V O}=0.33 imes 53.3=17.6 psi[/latex]

The shell-side pressure drop greatly exceeds the allowable value of 5 psi. Therefore, we consider increasing the baffle spacing. From Appendix 5.C, the maximum unsupported tube length for 3/4-in. copper alloy tubes is 52 in. Since the tubes in the baffle windows are supported by every other baffle, the maximum allowable baffle spacing is 26 in., which is less than the shell diameter. So in this case, the baffle spacing is limited by tube support considerations. When adjusted for an integral number of baffles, the maximum spacing is decreased to 24 in., and this is too small to reduce the pressure drop to the required level.
Next we consider using a split-flow (type J) shell. The inlet vapor stream is divided into two parts that are fed to opposite ends of the shell and flow toward the center. Since both the flow rate and length of the flow path are halved, the shell-side pressure drop is reduced by a factor of approximately eight compared with an E-shell of the same size. Therefore, for the third trial an AJU exchanger with a 39-in. shell, 1336 tubes and four tube passes is specified. The baffle spacing is decreased somewhat to provide better tube support with seven baffle spaces in each half of the shell. Thus,

[latex]B=\frac{16 imes 12}{14}=13.7 in[/latex]

The middle baffle is a full circle baffle, as shown in Figure 11.2. These changes have no effect on the calculations for [latex]h_{i}[/latex], [latex]\Delta P_{i}[/latex], and [latex]h_{0}[/latex]. However, F, [latex]h_{V}[/latex], and [latex]\Delta P_{0} [/latex] must be recalculated.

Third trial
(a) LMTD correction factor.
From the graph in Appendix 11.A for a J shell with an even number of tube passes, F ≅ 0.98, which is essentially the same as the value for the E shell. Therefore, the required overall coefficient remains unchanged at 86 Btu/h . ft².ºF.
(b) Correction for sensible heat transfer

[latex]a_{s}=\frac{d_{5} C^{\prime} B}{144 P_{T}}=\frac{39 imes 0.1875 imes 13.7}{144 imes 15 / 16}=0.742 ft ^{2}[/latex]

Half the average vapor flow rate of 90,000 lb/h is used to calculate [latex]h_{V}[/latex]. Thus,

[latex]G=\dot{m} / a_{s}=\frac{0.5 imes 90,000}{0.742}=60,647 lbm / h \cdot ft ^{2}[/latex]

[latex]R e=\frac{D_{e} G}{\mu_{V}}=\frac{0.04583 imes 60,647}{0.0085 imes 2.419}=135,177[/latex]

[latex]B / d_{s}=13.7 / 39=0.351[/latex]

[latex]j_{H}=0.5\left(1+B / d_{S} ight)\left(0.08 R e^{0.6821}+0.7 R e^{0.1772} ight)[/latex]

[latex]=0.5(1+0.351)\left\{0.08(135,177)^{0.6821}+0.7(135,177)^{0.1772} ight\}[/latex]

[latex]j_{H}=174.6[/latex]

[latex]h_{V}=j_{H}\left(k / D_{e} ight) P_{T}^{1 / 3}\left(\mu / \mu_{w} ight)^{0.14}[/latex]

[latex]h_{V}=174.6(0.0119 / 0.04583)(0.84)^{1 / 3}(1.0)[/latex]

[latex]h_{V} \cong 43 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

The modified overall coefficient then becomes:

[latex]\dot{U}_{D}=\left[U_{D}^{-1}+\left(q_{\operatorname{sen}} / q_{T o t} ight) h_{V}^{-1} ight]^{-1}=\left[(94)^{-1}+0.026(43)^{-1} ight][/latex]

[latex]\dot{U}_{D}=89 Btu / h \cdot ft ^{2} \cdot{ }^{\circ} F[/latex]

Since [latex]\dot{U}_{D} > U_{req}[/latex], the unit is thermally acceptable.
(c) Shell-side pressure drop.
Half the total mass flow rate of vapor entering the condenser is used to compute [latex]\left(\Delta P_{f} ight)_{V O}[/latex] for the J shell. Thus

[latex]G=0.5 \dot{m}_{V} / a_{5}=0.5 imes 180,000 / 0.742=121,294 lbm / h \cdot ft ^{2}[/latex]

[latex]R e=\frac{D_{e} G}{\mu_{V}}=\frac{0.04583 imes 121,294}{0.0085 imes 2.419}=270,355[/latex]

[latex]f_{1}=\left(0.0076+0.000166 d_{5} ight) R e^{-0.125}[/latex]

[latex]=(0.0076+0.000166 imes 39)(270,355)^{-0.125}[/latex]

[latex]f_{1}=0.00295 ft ^{2} / ext { in. }^{2}[/latex]

[latex]f_{2}=\left(0.0016+5.8 imes 10^{-5} d_{s} ight) R e^{-0.157}[/latex]

[latex]=\left(0.0016+5.8 imes 10^{-5} imes 23.25 ight)(270,355)^{-0.157}[/latex]

[latex]f_{2}=0.00041 ft ^{2} / ext { in. }^{2}[/latex]

[latex]f=144\left\{f_{1}-1.25\left(1-B / d_{5} ight)\left(f_{1}-f_{2} ight) ight\}[/latex]

[latex]=144\{0.00295-1.25(1-0.351)(0.00295-0.000414)\}[/latex]

f = 0.1285

[latex]\left(\Delta P_{f} ight)_{V O}=\frac{f G^{2} d_{s}\left(n_{b}+1 ight)}{7.50 imes 10^{12} D_{e} s \phi}=\frac{0.1285(121,294)^{2}(39 / 12)(7)}{7.50 imes 10^{12} imes 0.04583(0.845 / 62.43)(1.0)}[/latex]

[latex]\left(\Delta P_{f} ight)_{V O}=9.24 psi[/latex]

Notice that ([latex]n_{b}+1[/latex]) = 7 here, rather than 14, because the flow traverses only half the length of the J-shell.

[latex]\Delta P_{f}=\bar{\phi}_{V O}^{2}\left(\Delta P_{f} ight)_{V O}=0.33 imes 9.24=3.05 psi[/latex]

Assuming 10-in. schedule 40 nozzles for the vapor and allowing one velocity head for the inlet loss gives:

[latex]G_{n, ext { in }}=\frac{90,000}{(\pi / 4)(10.02 / 12)^{2}}=164,354 lbm / h \cdot ft ^{2}[/latex]

[latex]\Delta P_{n, ext { in }}=1.334 imes 10^{-13} G_{n, ext { in }}^{2} / s_{V}=\frac{1.334 imes 10^{-13}(164,354)^{2}}{(0.845 / 62.43)}[/latex]

[latex]\Delta P_{n, ext { in }}=0.266 psi[/latex]

The condensate nozzle will be sized for self-venting operation. The volumetric flow rate is:

[latex]\dot{v}_{ L }=\dot{m} / ho=(180,000 / 3600) / 35.5=1.408 ft ^{3} / s[/latex]

The minimum nozzle size is obtained from Inequality (11.5a):

[latex]D_{n}>0.89 \dot{v}_{L}^{0.4}=0.89(1.408)^{0.4}=1.02 ft =12.24 in[/latex]

Assuming schedule 40 pipe is used, the smallest size that satisfies this condition is 14-in. pipe (from Table B.2).
The pressure drop across the self-venting condensate nozzle will be very small and can be neglected. Hence, the total shell-side pressure drop is:

[latex]\Delta P_{o}=\Delta P_{f}+\Delta P_{n, ext { in }}=3.05+0.266 \cong 3.3 psi[/latex]

All design criteria are satisfied and the over-design is approximately 3.5%. Hence, the unit is acceptable as configured.
Final design summary
Tube-side fluid: cooling water
Shell-side fluid: condensing hydrocarbons
Shell: Type AJU, 39 in. ID, oriented horizontally
Number of tubes: 1336
Tube size: 0.75 in. OD, 16 BWG, 16-ft long
Tube layout: 15/16-in. triangular pitch
Tube passes: 4
Baffles: 35% cut segmental type with vertical cut; middle baffle is full circle
Baffle spacing: approximately 13.7 in.
Number of baffles: 13Sealing strips: as required based on detailed tube layout
Tube-side nozzles: 10-in. schedule 40 inlet and outlet
Shell-side nozzles: two 10-in. schedule 40 inlet (top), one 14-in. schedule 40 outlet (bottom)
Materials: Tubes and tubesheets, 90/10 copper-nickel alloy; all other components, carbon steel

Question 11.6: 180,000 lb/h of a saturated vapor consisting of 30 mole perc...

Related Answered Questions

Use Xist to obtain a final design for the C4–C5 condenser of Example 11.9. ...

Verified Answer:

Verified Answer:

Calculate the LMTD correction factor for the following cases: (a) A J-shell with one tube pass, R = 0.5 and P = 0.75.(b) A J-shell with two tube passes, R = 1.0 and P = 0.5. ...

Verified Answer:

Design a finned-tube condenser for the service of Example 11.6. ...

Verified Answer:

Verified Answer:

Use HEXTRAN and Xist to rate the final design for the C4–C5 condenser obtained in Example 11.6, and compare the results with those from the hand calculations. ...

Verified Answer:

Verified Answer:

A stream consisting of 5000 lb/h of saturated n-propyl alcohol (1-propanol) vapor at 207º F will be condensed using a tube bundle containing 109 tubes arranged for one pass. The tubes are 0.75 in. OD, 14 BWG, with a length of 12 ft. Estimate the condensing-side heat-transfer coefficient for ...

Verified Answer:

Evaluate the significance of interfacial shear for the conditions of Example 11.2 and the following specifications: (a) For the vertical condenser, the vapor flows downward through the tubes. (b) For the horizontal shell-side condenser, the unit consists of an E-shell with an ID of 12 in. The ...

Verified Answer:

Verified Answer: