Question 15.5: Determine the reactions at the supports for the prismatic 61...

Equilibrium Equations. From the free-body diagram of Fig. 15.23b we write

\underrightarrow{+} \sum{F_{x}} = 0:  A_{x} = 0

+↑\sum{F_{y}} = 0:   A_{y} + B – wL =0

+\curvearrowleft \sum{M_{A}} = 0:   M_{A} + BL – \frac{1}{2} wL^{2} = 0                      (15.38)

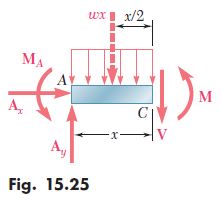
Equation of Elastic Curve. Drawing the free-body diagram of a portion of beam AC (Fig. 15.25), we write

+\curvearrowleft \sum{M_{C}} = 0:   M + \frac{1}{2}wx^{2} + M_{A} – A_{y} x = 0                                   (15.39)

Solving Eq. (15.39) for M and carrying into Eq. (15.4), we write

EI \frac{d^{2}y}{dx^{2}} =\frac{M(x)}{EI}
(15.4)

EI \frac{d^{2}y}{dx^{2}} = – \frac{1}{2} wx^{2} + A_{y} x – M_{A}

Integrating in x, we have

EI  θ = EI \frac{dy}{dx}= – \frac{1}{6} wx^{3} + \frac{1}{2} A_{y} x^{2} – M_{A}x + C_{1}                           (15.40)

EI  y = – \frac{1}{24} wx^{4} + \frac{1}{6} A_{y} x^{3} -\frac{1}{2} M_{A}x^{2} + C_{1}x + C_{2}                      (15.41)

ferring to the boundary conditions indicated in Fig. 15.24, we make x = 0, θ = 0 in Eq. (15.40), x = 0, y = 0 in Eq. (15.41), and conclude that C_{1} = C_{2} = 0. Thus, we rewrite Eq. (15.41) as follows:

EI  y = -\frac{1}{24} wx^{4} + \frac{1}{6} A_{y} x^{3} – \frac{1}{2} M_{A} x^{2}                             (15.42)

But the third boundary condition requires that y = 0 for x = L. Carrying these values into (15.42), we write

0 = -\frac{1}{24} wL^{4} + \frac{1}{6} A_{y} L^{3} – \frac{1}{2} M_{A}L^{2}

or

3M_{A} – A_{y} L + \frac{1}{4} wL^{2} = 0                 (15.43)

Solving this equation simultaneously with the three equilibrium equations (15.38), we obtain the reactions at the supports:

A_{x} = 0                  A_{y} = \frac{5}{8} wL                  M_{A} = \frac{1}{8} wL^{2}                 B = \frac{3}{8}wL