Question 10.13: Determining the Relationship Between Geometric Shapes and th...
Determining the Relationship Between Geometric Shapes and the Resultant Dipole Moments of Molecules
Which of these molecules would you expect to be polar: Cl_2, ICl, BF_3, NO, SO_2?
Analyze
We will use the methods described above to determine the shape of the molecule, and then ascertain whether or not bond dipoles, if present, produce a net permanent dipole moment.
Polar: ICl, NO, SO_2 . ICl and NO are diatomic molecules with an electronegativity difference between the bonded atoms. SO_2 is a bent molecule with an electronegativity difference between the S and O atoms.
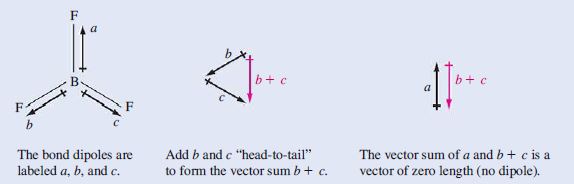
Nonpolar: Cl_2 and BF_3. Cl_2 is a diatomic molecule of identical atoms; hence no electronegativity difference. For BF_3, refer to Table 10.1. BF_3 is a symmetrical planar molecule (120° bond angles). The \begin{matrix} B-F \end{matrix} bond dipoles cancel each other.
| TABLE 10.1 Molecular Geometry as a Function of Electron-Group Geometry | ||||||
| Number of Electron Groups | Electron-Group Geometry | Number of Lone Pairs | VSEPR Notation | Molecular Geometry | Ideal Bond Angles | Example |
| 2 | linear | 0 | A \chi_2 |  |
180° | BeCl_2  |
| 3 | trigonal planar | 0 | A \chi_3 |  |
120° | BF_3  |
| trigonal planar | 1 | A \chi_2 E |  |
120° | SO_2{ }^a | |
| 4 | tetrahedral | 0 | A \chi_4 |  |
109.5° | CH_4  |
| tetrahedral | 1 | A \chi_3 E |  |
109.5° | NH_3 | |
| tetrahedral | 2 | A \chi_2 E_2 |  |
109.5° | OH_2 | |
| 5 | trigonal bipyramidal | 0 | A \chi_5 |  |
90°,120° | PCl_5  |
| trigonal bipyramidal | 1 | A \chi_4 E^b |  |
90°,120° | SF_4 | |
| trigonal bipyramidal | 2 | A \chi_3 E_2 |  |
90° | ClF_3 | |
| trigonal bipyramidal | 3 | A \chi_2 E_3 |  |
180° | \chi eF_2 | |
| 6 | octahedral | 0 | A \chi_6 |  |
90° | SF_6  |
| octahedral | 1 | A \chi_5 E |  |
90° | BrF_5 | |
| octahedral | 2 | A \chi_4 E_2 |  |
90° | \chi eF_4 | |
_{}^{a}\textrm{For }a discussion of the structure of SO_2, see page 428.
_{}^{b}\textrm{For }a discussion of the placement of the lone-pair electrons in this structure, see page 427.
Assess
Bond dipoles are vector quantities. When adding them together, we must add them as vectors, that is, “head-to-tail,” as illustrated below.
