Entropy Maximum Principle A 2 kg copper block at 100°C is brought in thermal contact with a 5 kg aluminum block at 50°C. Treating the combined system as an isolated system, determine the final temperature (a) by assuming that at equilibrium the temperatures of the two blocks are equal and (b) by

Question

Entropy Maximum Principle

A 2 kg copper block at 100°C is brought in thermal contact with a 5 kg aluminum block at 50°C. Treating the combined system as an isolated system, determine the final temperature (a) by assuming that at equilibrium the temperatures of the two blocks are equal and (b) by using the entropy maximum principle, without assuming the final temperatures to be equal.

Accepted Answer

Perform an energy and entropy analysis of the closed process executed by the combined non-uniform system within the red boundary of Figure 14.3.

Assumptions
The SL model is applicable to the subsystems and a composite state made of two local states in LTE can describe the global beginning and final states. No changes in KE or PE.

Analysis
The copper block is sub-system A and the aluminum block is sub-system B. From Table A-1, or any SL TESTcalc, we obtain [latex] c_{v A}=0.386 kJ / kg \cdot K ext { and } c_{v B}=0.90 kJ / kg \cdot K [/latex]. Designating the composite beginning state (bA and bB states) by States 1 and 2 and the composite final state ( fA and fB states) by States 3 and 4 (Fig. 14.3), the energy equation for the closed process, Eq. (5.2), can be simplified:

[latex]\underbrace{\Delta E=E_{f}-E_{b}}_{\begin{array}{l} ext { Change in } E ext { during } \ ext { the process. }\end{array}}=\underbrace{Q}_{\begin{array}{l} ext { Heat added to } \ ext { the system. }\end{array}}-\underbrace{W_{ ext {ext }}}_{\begin{array}{l} ext { Work transferred } \ ext { out of the system. }\end{array}} ; \quad[ kJ ][/latex] (5.2)

[latex]\begin{aligned}&\Delta U=\cancel{Q}^{0}-\cancel{W}^{0} ; \quad \Rightarrow \quad \Delta\left(U_{A}+U_{B} ight)=0 ; \quad \Rightarrow \quad \Delta U_{A}=-\Delta U_{B} ;\&\Rightarrow \quad m_{A} c_{v A}\left(T_{f A}-T_{b A} ight)=m_{B} c_{v B}\left(T_{b B}-T_{f B} ight) ext {; }\&\Rightarrow \quad m_{A} c_{v A}\left(T_{3}-T_{1} ight)=m_{B} c_{v B}\left(T_{2}-T_{4} ight)\end{aligned}[/latex]

Substituting the known variables, the energy equation reduces to:

[latex]T_{3}=2256-5.83 T_{4}[/latex] (14.3)

Given (and also known from our experience) that [latex] T_{4}=T_{3}=T_{f} [/latex] , Eq. (14.3) produces [latex] T_{f} [/latex] as 330.3 K or 57.3°C.

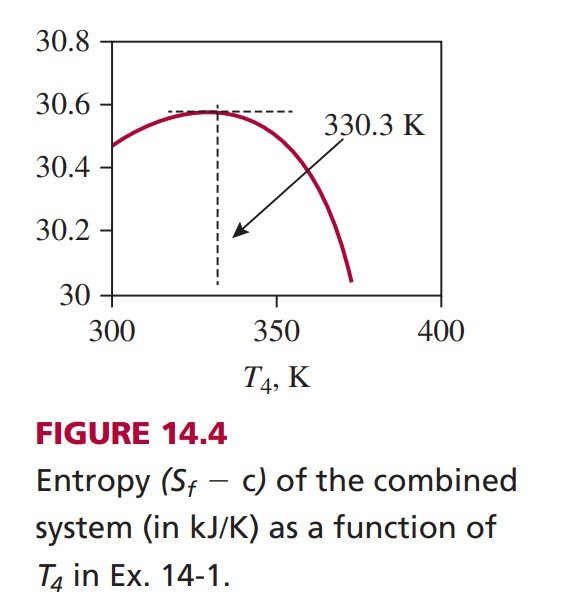
(b) The entropy of the combined system, which is isolated, reaches a maximum at equilibrium. To locate the maximum, one approach is to plot the entropy of the combined system against all possible final states. The entropy of the composite system can be expressed in terms of [latex] T_{3} ext { and } T_{4} [/latex] using the SL model:

[latex]\begin{aligned}&S_{f}-S_{b}=\left(S_{f A}+S_{f B} ight)-\left(S_{b A}+S_{b B} ight) \&\Rightarrow \quad \Delta S=\left(S_{f A}-S_{b A} ight)+\left(S_{f B}-S_{b B} ight)=m_{A} c_{v A} \ln \frac{T_{f A}}{T_{b A}}+m_{B} c_{v B} \ln \frac{T_{f B}}{T_{b B}} \&\Rightarrow \quad S_{f}=\left(S_{b}-m_{A} c_{v A} \ln T_{1}-m_{B} c_{v B} \ln T_{2} ight)+m_{A} c_{v A} \ln T_{3}+m_{B} c_{v B} \ln T_{4}\end{aligned}[/latex]

Representing the terms inside the parentheses by a constant c, and substituting the constraint from the energy equation, Eq. (14.3), the final entropy can be expressed as a function of [latex] T_{4} [/latex] alone:

[latex] S_{f}= c +0.772 \ln \left(2256-5.83 T_{4} ight)+4.5 \ln T_{4} \quad [kJ/K][/latex] (14.4)

([latex] S_{f} [/latex] - c), evaluated from this expression, is plotted against [latex] T_{4} [/latex] (which represents the possible final temperature of sub-system B) in Figure 14.4. To obtain the precise temperature at which the entropy peaks, set the derivative of [latex] S_{f} [/latex] , with respect to [latex] T_{4} [/latex], to zero and solve the resulting equation to show that [latex] T_{4}=57.3^{\circ} C [/latex] at the maximum. This result is consistent with the maximum exhibited by the graph in Figure 14.4.

TEST Analysis
Launch the SL/SL non-uniform, non-mixing, closed-process TESTcalc. Evaluate State-1 and State-2 with the given information (mass and temperature) and leave the temperatures unknown for State-3 and State-4. For each state, make sure that the working substance is correctly selected (there are two working substance selectors, the left one can be used for sub-system A and the other one for sub-system B). In the process panel, set up the adiabatic process by selecting the composite anchor states and entering process variables Q=W_ext=0. Now guess a value of T3, say, 75 deg-C, and click Super-Calculate. The entropy change of the system, Delta_S, is calculated as 0.0058 kJ/K. Change T3 to a new value and Super-Calculate to update the value of Delta_S. It can be verified that when T3 is 57.3 deg-C, entropy change reaches a maximum, and T4 becomes equal to T3. See TEST-code (TEST > TEST-codes) for further details.

Discussion
If the two subsystems consist of two gases separated by a sliding piston, instead of two solid blocks, the entropy maximum principle leads to equality of pressure as well (see Anim. 14.A. mechEquilibrium). While the equality of temperature or pressure among subsystems at equilibrium is almost trivial, our experience falls far short in predicting more challenging equilibrium conditions when there is a phase or chemical transformation involved.

Question 14.1: Entropy Maximum Principle A 2 kg copper block at 100°C is br...

Related Answered Questions

Verified Answer:

Use of van’t Hoff Equation (a) Use van’t Hoff equation to estimate the heat of combustion for the reaction CO + 0.5O2 → CO2 at 2000 K. (b) Use TEST to verify the result. ...

Verified Answer:

Equilibrium Composition One kmol of hydrogen and one kmol of oxygen react to produce an equilibrium mixture of water, hydrogen, and oxygen at 2500 K. (a) Determine the equilibrium molar composition if the mixture pressure is 1 atm. What-if scenario: What would the mole fraction of water in ...

Verified Answer:

Equilibrium of an Ideal Gas Mixture A natural gas reservoir contains a mixture of methane and nitrogen at 298 K. At the top of the well, the mixture pressure is 100 kPa and the molar composition is found to be 50% methane and 50% nitrogen. Determine (a) the pressure and (b) the molar composition ...

Verified Answer:

Ionization and Neutral Component A mixture containing 95, helium and 5, cesium on molar basis is heated to 2000 K. The equilibrium constants for ionization of helium and cesium are 5000 and 15.63, respectively. Determine the pressure at which the mole fraction of electron’s is 0.01. ...

Verified Answer:

Verified Answer:

Equilibrium Constant Evaluate ln K at 298 K for the following elementary steps: (a) H2 + 0.5O2 S H2O and (b) 2H2O S 2H2 + O2. Assume water to be in the vapor phase. What-if scenario: What would the answer in part (a) be if the temperature was (c) 1000 K or (d) 2500 K? ...

Verified Answer:

Verified Answer:

Desalination through Reverse Osmosis (a) Determine the minimum useful work required for desalination per unit mass of ocean water at 10°C and 1 atm through reverse osmosis. (b) Compare it with the energy required for desalination by distillation. (c) Also calculate the osmotic head. Use ...

Verified Answer:

Verified Answer: