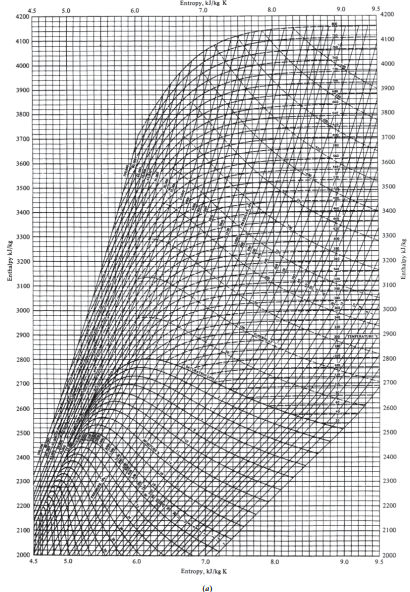
Another Example of Using the Entropy Balance in Problem Solving A steam turbine operates at the following conditions:a. Compute the horsepower developed by the turbine and the entropy change of the steam. b. Suppose the turbine is replaced with one that is well insulated, so that the heat loss is

Question

Another Example of Using the Entropy Balance in Problem Solving

A steam turbine operates at the following conditions:

[latex]\begin{array}{lccc}\hline & ext { Inlet } & & ext { Outlet } \\hline ext { Velocity }( m / min ) & 2000 & & 7500 \T( K ) & 800 & & 440 \P( MPa ) & 3.5 & & 0.15 \ ext { Flow rate }( kg / hr ) & & 10000 & \ ext { Heat loss }( kJ / hr ) & & 125000 & \\hline\end{array}[/latex]

a. Compute the horsepower developed by the turbine and the entropy change of the steam.

b. Suppose the turbine is replaced with one that is well insulated, so that the heat loss is eliminated, and well designed, so that the expansion is reversible. If the exit pressure and velocity are maintained at the previous values, what are the outlet steam temperature and the horsepower developed by the turbine?

Accepted Answer

The steady-state mass and energy balances on the turbine and its contents (the system) yield

[latex]\begin{gathered}\frac{d M}{d t}=0=\dot{M}_{1}+\dot{M}_{2} \quad \dot{M}_{2}=-\dot{M}_{1}=-10000 kg / hr \\frac{d}{d t}\left[U+M\left(\frac{\upsilon ^{2}}{2}+g h ight) ight]=0=\dot{M}_{1}\left(\hat{H}_{1}+\frac{\upsilon _{1}^{2}}{2} ight)+\dot{M}_{2}\left(\hat{H}_{2}+\frac{\upsilon _{2}^{2}}{2} ight)+\dot{W}_{s}+\dot{Q}\end{gathered}[/latex]

a. From the Mollier diagram of Fig. 3.3-1 (or Appendix A.III),

[latex]\hat{H}_{1} \simeq 3510 J / g[/latex]

and

[latex]\hat{S}_{1} \simeq 7.23 J /( g K )[/latex]

Also,

[latex]\frac{\upsilon _{1}^{2}}{2}=\frac{1}{2}\left(\frac{2000 m / min ^{2}}{60 s / min } ight)^{2} imes \frac{1 J / kg }{ m ^{2} / s ^{2}} imes \frac{1 kg }{1000 g }=0.56 J / g[/latex]

so that

[latex]\hat{H}_{1}+\frac{\upsilon _{1}^{2}}{2}=3510.6 J / g[/latex]

Similarly,

[latex]\hat{H}_{2} \simeq 2805 J / g \quad \hat{H}_{2}+\frac{\upsilon _{2}^{2}}{2}=2805+7.8=2812.8 J / g[/latex]

and

[latex]\hat{S}_{2} \simeq 7.50 J /( g K )[/latex]

Therefore,

[latex]\begin{aligned}-\dot{W}_{s} &=(3510.6-2812.8) \frac{ J }{ g } imes 10000 \frac{ kg }{ hr } imes 1000 \frac{ g }{ kg }-12.5 imes 10^{4} \frac{ kJ }{ hr } imes 1000 \frac{ J }{ kJ } \&=6.853 imes 10^{9} \frac{ J }{ hr } imes \frac{1 kJ }{1000 J } imes \frac{1 hr }{3600 s }=1903.6 kJ / s =1.9036 imes 10^{6} W \&=2553 hp\end{aligned}[/latex]

Also,

[latex]\Delta \hat{S}=\left(\hat{S}_{2}-\hat{S}_{1} ight)=0.27 J /( g K )[/latex]

b. The steady-state entropy balance for the turbine and its contents is

[latex]\frac{d S}{d t}=0=\dot{M}_{1} \hat{S}_{1}-\dot{M}_{1} \hat{S}_{2}+\dot{S}_{ ext {gen }}[/latex]

since [latex]\dot{Q}=0, ext { and } \dot{M}_{2}=-\dot{M}_{1} . ext { Also, the turbine operates reversibly so that } \dot{S}_{ ext {gen }}=0[/latex], and [latex]\hat{S}_{1}=\hat{S}_{2}[/latex]; that is, the expansion is isentropic. We now use Fig. 3.3-1, the entropy-enthalpy plot (Mollier diagram) for steam, to solve this problem. In particular, we locate the initial steam conditions (T = 800 K, P = 3.5 MPa) on the chart and follow a line of constant entropy (a vertical line on the Mollier diagram) to the exit pressure (0.15 MPa), to obtain the enthalpy of the exiting steam [latex]\left(\hat{H}_{2} \approx 2690 J / g ight)[/latex] and its final temperature (T ≈ 373 K). Since the exit velocity is known, we can immediately compute the horsepower generated by the turbine:

[latex]\begin{aligned}-\dot{W}_{s} &=[(3510+0.6)-(2690+7.8)] \frac{ J }{ g } imes 10000 \frac{ kg }{ hr } imes \frac{1000 g / kg }{1000 J / kJ } imes \frac{1 hr }{3600 s } \&=2257.8 kJ / s =2.2578 imes 10^{6} W =3028 hp\end{aligned}[/latex]

Comments
1. Here, as before, the kinetic energy term is of negligible importance compared with the internal energy term.
2. Notice from the Mollier diagram that the turbine exit steam is right at the boundary of a two-phase mixture of vapor and liquid. For the solution of this problem, no difficulties arise if the exit steam is a vapor, a liquid, or a two-phase vapor-liquid mixture since our mass, energy, and entropy balances are of general applicability. In particular, the information required to use these balance equations is the internal energy, enthalpy, and entropy per unit mass of each of the flow streams. Provided we have this information, the balance equations can be used independent of whether the flow streams consist of single or multiple phases, or, in fact, single or multiple components. Here the Mollier diagram provides the necessary thermodynamic information, and the solution of this problem is straightforward.
3. Finally, note that more work is obtained from the turbine by operating it in a reversible and adiabatic manner.

Question 4.5-7: Another Example of Using the Entropy Balance in Problem Solv...

Related Answered Questions