Question 7.7: DAYTONA INTERNATIONAL SPEEDWAY GOAL Solve a centripetal forc...
DAYTONA INTERNATIONAL SPEEDWAY
GOAL Solve a centripetal force problem involving two dimensions.
PROBLEM The Daytona International Speedway in Daytona Beach, Florida, is famous for its races, especially the Daytona 500, held every February. Both of its courses feature four-story, 31.0° banked curves, with maximum radius of 316 m. If a car negotiates the curve too slowly, it tends to slip down the incline of the turn, whereas if it’s going too fast, it may begin to slide up the incline. (a) Find the necessary centripetal acceleration on this banked curve so the car won’t tend to slip down or slide up the incline. (Neglect friction.) (b) Calculate the speed of the race car.
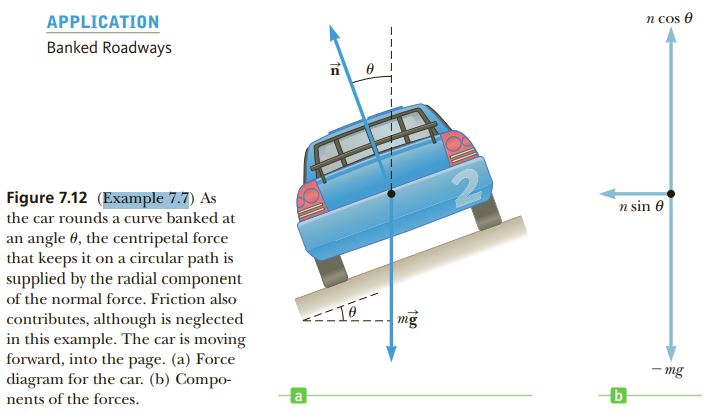
STRATEGY Two forces act on the race car: the force of gravity and the normal force \overrightarrow{\mathbf{n}}. (See Fig. 7.12.) Use Newton’s second law in the upward and radial directions to find the centripetal acceleration a_c Solving a_c=-v^2 / r for v then gives the race car’s speed.
Learn more on how we answer questions.
(a) Find the centripetal acceleration.
Write Newton’s second law for the car:
m \overrightarrow{\mathbf{a}}=\sum \overrightarrow{\mathbf{F}}=\overrightarrow{\mathbf{n}}+m \overrightarrow{\mathbf{g}}
Use the y-component of Newton’s second law to solve for the normal force n :
\begin{aligned}n \cos \theta-m g &=0 \\n &=\frac{m g}{\cos \theta}\end{aligned}
Obtain an expression for the horizontal component of \overrightarrow{\mathbf{n}}, which is the centripetal force F_c in this example:
F_c=-n \sin \theta=-\frac{m g \sin \theta}{\cos \theta}=-m g \tan \theta
Substitute this expression for F_c into the radial component of Newton’s second law and divide by m to get the centripetal acceleration:
\begin{aligned}&m a_c=F_c \\&a_c=\frac{F_c}{m}=-\frac{m g \tan \theta}{m}=-g \tan \theta \\&a_c=-\left(9.80 \mathrm{~m} / \mathrm{s}^2\right)\left(\tan 31.0^{\circ}\right)=-5.89 \mathrm{~m} / \mathrm{s}^2\end{aligned}
(b) Find the speed of the race car.
Apply Equation 7.13:
a_c = \frac{v^2}{r} [7.13]
\begin{aligned}&-\frac{v^2}{r}=a_c \\&v=\sqrt{-r a_c}=\sqrt{-(316 \mathrm{~m})\left(-5.89 \mathrm{~m} / \mathrm{s}^2\right)}=43.1 \mathrm{~m} / \mathrm{s}^2\end{aligned}
REMARKS In fact, both banking and friction assist in keeping the race car on the track.